Solving inequalities online

Before solving inequalities, it is necessary to understand well how equations are solved.

It doesn’t matter whether the inequality is strict () or non-strict (≤, ≥), the first step is to solve the equation by replacing the inequality sign with equality (=).

Explain what it means to solve an inequality?

After studying the equations, the student has the following picture in his head: you need to find such values ​​of the variable for which both parts of the equation take the same values. In other words, find all points where the equality holds. Everything is correct!

When talking about inequalities, they mean finding the intervals (segments) on which the inequality holds. If there are two variables in the inequality, then the solution will no longer be intervals, but some areas on the plane. Guess what will be the solution of the inequality in three variables?

How to solve inequalities?

The method of intervals (aka the method of intervals) is considered to be a universal way to solve inequalities, which consists in determining all the intervals within which the given inequality will be fulfilled.

Without going into the type of inequality, in this case it is not the essence, it is required to solve the corresponding equation and determine its roots, followed by the designation of these solutions on the numerical axis.

What is the correct way to write the solution to an inequality?

When you have determined the intervals for solving the inequality, you need to correctly write out the solution itself. There is an important nuance - are the boundaries of the intervals included in the solution?

Everything is simple here. If the solution of the equation satisfies the ODZ and the inequality is not strict, then the boundary of the interval is included in the solution of the inequality. Otherwise, no.

Considering each interval, the solution to the inequality can be the interval itself, or a half-interval (when one of its boundaries satisfies the inequality), or a segment - an interval together with its boundaries.

Important point

Do not think that only intervals, half-intervals and segments can be the solution to an inequality. No, individual points can also be included in the solution.

For example, the inequality |x|≤0 has only one solution - point 0.

And the inequality |x|

What is the inequality calculator for?

The inequality calculator gives the correct final answer. In this case, in most cases, an illustration of a numerical axis or plane is given. You can see whether the boundaries of the intervals are included in the solution or not - the points are displayed filled or pierced.

Thanks to the online inequality calculator, you can check whether you have found the roots of the equation correctly, marked them on the number line and checked the inequality conditions on the intervals (and boundaries)?

If your answer differs from the answer of the calculator, then you definitely need to double-check your solution and identify the mistake made.

Today, friends, there will be no snot and sentiment. Instead, I will send you into battle with one of the most formidable opponents in the 8th-9th grade algebra course without further questions.

Yes, you understood everything correctly: we are talking about inequalities with a modulus. We will look at four basic techniques with which you will learn to solve about 90% of these problems. What about the other 10%? Well, we'll talk about them in a separate lesson. :)

However, before analyzing any tricks there, I would like to recall two facts that you already need to know. Otherwise, you risk not understanding the material of today's lesson at all.

What you already need to know

Captain Evidence, as it were, hints that in order to solve inequalities with a modulus, you need to know two things:

1. How are inequalities resolved?
2. What is a module.

Let's start with the second point.

Module Definition

Everything is simple here. There are two definitions: algebraic and graphic. Let's start with the algebra:

Definition. The module of the number $x$ is either the number itself, if it is non-negative, or the number opposite to it, if the original $x$ is still negative.

It is written like this:

\[\left| x \right|=\left\( \begin(align) & x,\ x\ge 0, \\ & -x,\ x \lt 0. \\\end(align) \right.\]

In simple terms, the modulus is “a number without a minus”. And it is in this duality (somewhere you don’t need to do anything with the original number, but somewhere you have to remove some minus there) and all the difficulty for novice students lies.

There is also a geometric definition. It is also useful to know it, but we will refer to it only in complex and some special cases, where the geometric approach is more convenient than the algebraic one (spoiler: not today).

Definition. Let the point $a$ be marked on the real line. Then the module $\left| x-a \right|$ is the distance from the point $x$ to the point $a$ on this line.

If you draw a picture, you get something like this:

One way or another, its key property immediately follows from the definition of the module: the modulus of a number is always a non-negative value. This fact will be a red thread running through our entire story today.

Solution of inequalities. Spacing Method

Now let's deal with inequalities. There are a great many of them, but our task now is to be able to solve at least the simplest of them. Those that are reduced to linear inequalities, as well as to the method of intervals.

I have two big tutorials on this topic (by the way, very, VERY useful - I recommend studying):

1. The interval method for inequalities (especially watch the video);
2. Fractional-rational inequalities is a very voluminous lesson, but after it you will not have any questions left at all.

If you know all this, if the phrase "let's move from inequality to equation" does not make you vaguely want to kill yourself against the wall, then you are ready: welcome to hell to the main topic of the lesson. :)

1. Inequalities of the form "Module less than function"

This is one of the most frequently encountered tasks with modules. It is required to solve an inequality of the form:

\[\left| f\right| \ltg\]

Anything can act as functions $f$ and $g$, but usually they are polynomials. Examples of such inequalities:

\[\begin(align) & \left| 2x+3\right| \ltx+7; \\ & \left| ((x)^(2))+2x-3 \right|+3\left(x+1 \right) \lt 0; \\ & \left| ((x)^(2))-2\left| x \right|-3 \right| \lt 2. \\\end(align)\]

All of them are solved literally in one line according to the scheme:

\[\left| f\right| \lt g\Rightarrow -g \lt f \lt g\quad \left(\Rightarrow \left\( \begin(align) & f \lt g, \\ & f \gt -g \\\end(align) \right.\right)\]

It is easy to see that we get rid of the module, but instead we get a double inequality (or, which is the same thing, a system of two inequalities). But this transition takes into account absolutely all possible problems: if the number under the module is positive, the method works; if negative, it still works; and even with the most inadequate function in place of $f$ or $g$, the method will still work.

Naturally, the question arises: is it not easier? Unfortunately, you can't. This is the whole point of the module.

But enough of the philosophizing. Let's solve a couple of problems:

Task. Solve the inequality:

\[\left| 2x+3\right| \ltx+7\]

Decision. So, we have a classical inequality of the form “the module is less than” - there is even nothing to transform. We work according to the algorithm:

\[\begin(align) & \left| f\right| \lt g\Rightarrow -g \lt f \lt g; \\ & \left| 2x+3\right| \lt x+7\Rightarrow -\left(x+7 \right) \lt 2x+3 \lt x+7 \\\end(align)\]

Do not rush to open the brackets that are preceded by a “minus”: it is quite possible that because of the haste you will make an offensive mistake.

\[-x-7 \lt 2x+3 \lt x+7\]

\[\left\( \begin(align) & -x-7 \lt 2x+3 \\ & 2x+3 \lt x+7 \\ \end(align) \right.\]

\[\left\( \begin(align) & -3x \lt 10 \\ & x \lt 4 \\ \end(align) \right.\]

\[\left\( \begin(align) & x \gt -\frac(10)(3) \\ & x \lt 4 \\ \end(align) \right.\]

The problem has been reduced to two elementary inequalities. We note their solutions on parallel real lines:

Intersection of many

The intersection of these sets will be the answer.

Answer: $x\in \left(-\frac(10)(3);4 \right)$

Task. Solve the inequality:

\[\left| ((x)^(2))+2x-3 \right|+3\left(x+1 \right) \lt 0\]

Decision. This task is a little more difficult. To begin with, we isolate the module by moving the second term to the right:

\[\left| ((x)^(2))+2x-3 \right| \lt -3\left(x+1 \right)\]

Obviously, we again have an inequality of the form “the module is less”, so we get rid of the module according to the already known algorithm:

\[-\left(-3\left(x+1 \right) \right) \lt ((x)^(2))+2x-3 \lt -3\left(x+1 \right)\]

Now attention: someone will say that I'm a bit of a pervert with all these brackets. But once again I remind you that our key goal is correctly solve the inequality and get the answer. Later, when you have perfectly mastered everything that is described in this lesson, you can pervert yourself as you like: open brackets, add minuses, etc.

And for starters, we just get rid of the double minus on the left:

\[-\left(-3\left(x+1 \right) \right)=\left(-1 \right)\cdot \left(-3 \right)\cdot \left(x+1 \right) =3\left(x+1\right)\]

Now let's open all the brackets in the double inequality:

Let's move on to double inequality. This time the calculations will be more serious:

\[\left\( \begin(align) & ((x)^(2))+2x-3 \lt -3x-3 \\ & 3x+3 \lt ((x)^(2))+2x -3 \\ \end(align) \right.\]

\[\left\( \begin(align) & ((x)^(2))+5x \lt 0 \\ & ((x)^(2))-x-6 \gt 0 \\ \end( align)\right.\]

Both inequalities are square and are solved by the interval method (that's why I say: if you don't know what it is, it's better not to take on the modules yet). We pass to the equation in the first inequality:

\[\begin(align) & ((x)^(2))+5x=0; \\ & x\left(x+5 \right)=0; \\ & ((x)_(1))=0;((x)_(2))=-5. \\\end(align)\]

As you can see, the output turned out to be an incomplete quadratic equation, which is solved elementarily. Now let's deal with the second inequality of the system. There you have to apply Vieta's theorem:

\[\begin(align) & ((x)^(2))-x-6=0; \\ & \left(x-3 \right)\left(x+2 \right)=0; \\& ((x)_(1))=3;((x)_(2))=-2. \\\end(align)\]

We mark the obtained numbers on two parallel lines (separate for the first inequality and separate for the second):

Again, since we are solving a system of inequalities, we are interested in the intersection of the shaded sets: $x\in \left(-5;-2 \right)$. This is the answer.

Answer: $x\in \left(-5;-2 \right)$

I think after these examples the solution scheme is very clear:

1. Isolate the module by moving all other terms to the opposite side of the inequality. Thus we get an inequality of the form $\left| f\right| \ltg$.
2. Solve this inequality by getting rid of the module as described above. At some point, it will be necessary to move from a double inequality to a system of two independent expressions, each of which can already be solved separately.
3. Finally, it remains only to cross the solutions of these two independent expressions - and that's it, we will get the final answer.

A similar algorithm exists for inequalities of the following type, when the modulus is greater than the function. However, there are a couple of serious "buts". We will talk about these “buts” now.

2. Inequalities of the form "Module is greater than function"

They look like this:

\[\left| f\right| \gt g\]

Similar to the previous one? Seem to be. Nevertheless, such tasks are solved in a completely different way. Formally, the scheme is as follows:

\[\left| f\right| \gt g\Rightarrow \left[ \begin(align) & f \gt g, \\ & f \lt -g \\\end(align) \right.\]

In other words, we consider two cases:

1. First, we simply ignore the module - we solve the usual inequality;
2. Then, in fact, we open the module with the minus sign, and then we multiply both parts of the inequality by −1, with a sign.

In this case, the options are combined with a square bracket, i.e. We have a combination of two requirements.

Pay attention again: before us is not a system, but an aggregate, therefore in the answer, the sets are combined, not intersected. This is a fundamental difference from the previous paragraph!

In general, many students have a lot of confusion with unions and intersections, so let's look into this issue once and for all:

• "∪" is a concatenation sign. In fact, this is a stylized letter "U", which came to us from the English language and is an abbreviation for "Union", i.e. "Associations".
• "∩" is the intersection sign. This crap didn't come from anywhere, but just appeared as an opposition to "∪".

To make it even easier to remember, just add legs to these signs to make glasses (just don’t accuse me of promoting drug addiction and alcoholism now: if you are seriously studying this lesson, then you are already a drug addict):

Translated into Russian, this means the following: the union (collection) includes elements from both sets, therefore, no less than each of them; but the intersection (system) includes only those elements that are both in the first set and in the second. Therefore, the intersection of sets is never greater than the source sets.

So it became clearer? That is great. Let's move on to practice.

Task. Solve the inequality:

\[\left| 3x+1 \right| \gt 5-4x\]

Decision. We act according to the scheme:

\[\left| 3x+1 \right| \gt 5-4x\Rightarrow \left[ \begin(align) & 3x+1 \gt 5-4x \\ & 3x+1 \lt -\left(5-4x \right) \\\end(align) \ right.\]

We solve each population inequality:

\[\left[ \begin(align) & 3x+4x \gt 5-1 \\ & 3x-4x \lt -5-1 \\ \end(align) \right.\]

\[\left[ \begin(align) & 7x \gt 4 \\ & -x \lt -6 \\ \end(align) \right.\]

\[\left[ \begin(align) & x \gt 4/7\ \\ & x \gt 6 \\ \end(align) \right.\]

We mark each resulting set on the number line, and then combine them:

Union of sets

Obviously the answer is $x\in \left(\frac(4)(7);+\infty \right)$

Answer: $x\in \left(\frac(4)(7);+\infty \right)$

Task. Solve the inequality:

\[\left| ((x)^(2))+2x-3 \right| \gtx\]

Decision. Well? No, it's all the same. We pass from an inequality with a modulus to a set of two inequalities:

\[\left| ((x)^(2))+2x-3 \right| \gt x\Rightarrow \left[ \begin(align) & ((x)^(2))+2x-3 \gt x \\ & ((x)^(2))+2x-3 \lt -x \\\end(align) \right.\]

We solve each inequality. Unfortunately, the roots will not be very good there:

\[\begin(align) & ((x)^(2))+2x-3 \gt x; \\ & ((x)^(2))+x-3 \gt 0; \\ &D=1+12=13; \\ & x=\frac(-1\pm \sqrt(13))(2). \\\end(align)\]

In the second inequality, there is also a bit of game:

\[\begin(align) & ((x)^(2))+2x-3 \lt -x; \\ & ((x)^(2))+3x-3 \lt 0; \\ &D=9+12=21; \\ & x=\frac(-3\pm \sqrt(21))(2). \\\end(align)\]

Now we need to mark these numbers on two axes - one axis for each inequality. However, you need to mark the points in the correct order: the larger the number, the further the point shifts to the right.

And here we are waiting for a setup. If everything is clear with the numbers $\frac(-3-\sqrt(21))(2) \lt \frac(-1-\sqrt(13))(2)$ (the terms in the numerator of the first fraction are less than the terms in the numerator of the second , so the sum is also smaller), with the numbers $\frac(-3-\sqrt(13))(2) \lt \frac(-1+\sqrt(21))(2)$ there will also be no difficulty (a positive number obviously more negative), but with the last couple, everything is not so simple. Which is larger: $\frac(-3+\sqrt(21))(2)$ or $\frac(-1+\sqrt(13))(2)$? The arrangement of points on the number lines and, in fact, the answer will depend on the answer to this question.

So let's compare:

\[\begin(matrix) \frac(-1+\sqrt(13))(2)\vee \frac(-3+\sqrt(21))(2) \\ -1+\sqrt(13)\ vee -3+\sqrt(21) \\ 2+\sqrt(13)\vee \sqrt(21) \\\end(matrix)\]

We isolated the root, got non-negative numbers on both sides of the inequality, so we have the right to square both sides:

\[\begin(matrix) ((\left(2+\sqrt(13) \right))^(2))\vee ((\left(\sqrt(21) \right))^(2)) \ \4+4\sqrt(13)+13\vee 21 \\ 4\sqrt(13)\vee 3 \\\end(matrix)\]

I think it's a no brainer that $4\sqrt(13) \gt 3$, so $\frac(-1+\sqrt(13))(2) \gt \frac(-3+\sqrt(21)) (2)$, finally the points on the axes will be arranged like this:

Case of ugly roots

Let me remind you that we are solving a set, so the answer will be the union, and not the intersection of the shaded sets.

Answer: $x\in \left(-\infty ;\frac(-3+\sqrt(21))(2) \right)\bigcup \left(\frac(-1+\sqrt(13))(2 );+\infty\right)$

As you can see, our scheme works great both for simple tasks and for very hard ones. The only “weak spot” in this approach is that you need to correctly compare irrational numbers (and believe me: these are not only roots). But a separate (and very serious lesson) will be devoted to questions of comparison. And we move on.

3. Inequalities with non-negative "tails"

So we got to the most interesting. These are inequalities of the form:

\[\left| f\right| \gt\left| g\right|\]

Generally speaking, the algorithm that we are going to talk about now is true only for the module. It works in all inequalities where there are guaranteed non-negative expressions on the left and right:

What to do with these tasks? Just remember:

In inequalities with non-negative tails, both sides can be raised to any natural power. There will be no additional restrictions.

First of all, we will be interested in squaring - it burns modules and roots:

\[\begin(align) & ((\left(\left| f \right| \right))^(2))=((f)^(2)); \\ & ((\left(\sqrt(f) \right))^(2))=f. \\\end(align)\]

Just do not confuse this with taking the root of the square:

\[\sqrt(((f)^(2)))=\left| f \right|\ne f\]

Countless mistakes were made when a student forgot to install a module! But this is a completely different story (these are, as it were, irrational equations), so we will not go into it now. Let's better solve a couple of problems:

Task. Solve the inequality:

\[\left| x+2 \right|\ge \left| 1-2x \right|\]

Decision. We immediately notice two things:

1. This is a non-strict inequality. Points on the number line will be punched out.
2. Both sides of the inequality are obviously non-negative (this is a property of the module: $\left| f\left(x \right) \right|\ge 0$).

Therefore, we can square both sides of the inequality to get rid of the modulus and solve the problem using the usual interval method:

\[\begin(align) & ((\left(\left| x+2 \right| \right))^(2))\ge ((\left(\left| 1-2x \right| \right) )^(2)); \\ & ((\left(x+2 \right))^(2))\ge ((\left(2x-1 \right))^(2)). \\\end(align)\]

At the last step, I cheated a little: I changed the sequence of terms, using the parity of the modulus (in fact, I multiplied the expression $1-2x$ by −1).

\[\begin(align) & ((\left(2x-1 \right))^(2))-((\left(x+2 \right))^(2))\le 0; \\ & \left(\left(2x-1 \right)-\left(x+2 \right) \right)\cdot \left(\left(2x-1 \right)+\left(x+2 \ right)\right)\le 0; \\ & \left(2x-1-x-2 \right)\cdot \left(2x-1+x+2 \right)\le 0; \\ & \left(x-3 \right)\cdot \left(3x+1 \right)\le 0. \\\end(align)\]

We solve by the interval method. Let's move from inequality to equation:

\[\begin(align) & \left(x-3 \right)\left(3x+1 \right)=0; \\ & ((x)_(1))=3;((x)_(2))=-\frac(1)(3). \\\end(align)\]

We mark the found roots on the number line. Once again: all points are shaded because the original inequality is not strict!

Getting rid of the module sign

Let me remind you for the especially stubborn: we take the signs from the last inequality, which was written down before moving on to the equation. And we paint over the areas required in the same inequality. In our case, this is $\left(x-3 \right)\left(3x+1 \right)\le 0$.

That's it. Problem solved.

Answer: $x\in \left[ -\frac(1)(3);3 \right]$.

Task. Solve the inequality:

\[\left| ((x)^(2))+x+1 \right|\le \left| ((x)^(2))+3x+4 \right|\]

Decision. We do everything the same. I will not comment - just look at the sequence of actions.

Let's square it:

\[\begin(align) & ((\left(\left| ((x)^(2))+x+1 \right| \right))^(2))\le ((\left(\left | ((x)^(2))+3x+4 \right| \right))^(2)); \\ & ((\left(((x)^(2))+x+1 \right))^(2))\le ((\left(((x)^(2))+3x+4 \right))^(2)); \\ & ((\left(((x)^(2))+x+1 \right))^(2))-((\left(((x)^(2))+3x+4 \ right))^(2))\le 0; \\ & \left(((x)^(2))+x+1-((x)^(2))-3x-4 \right)\times \\ & \times \left(((x) ^(2))+x+1+((x)^(2))+3x+4 \right)\le 0; \\ & \left(-2x-3 \right)\left(2((x)^(2))+4x+5 \right)\le 0. \\\end(align)\]

Spacing method:

\[\begin(align) & \left(-2x-3 \right)\left(2((x)^(2))+4x+5 \right)=0 \\ & -2x-3=0\ Rightarrow x=-1.5; \\ & 2((x)^(2))+4x+5=0\Rightarrow D=16-40 \lt 0\Rightarrow \varnothing . \\\end(align)\]

There is only one root on the number line:

The answer is a whole range

Answer: $x\in \left[ -1.5;+\infty \right)$.

A small note about the last task. As one of my students accurately noted, both submodule expressions in this inequality are obviously positive, so the modulus sign can be omitted without harm to health.

But this is already a completely different level of thinking and a different approach - it can be conditionally called the method of consequences. About him - in a separate lesson. And now let's move on to the final part of today's lesson and consider a universal algorithm that always works. Even when all previous approaches were powerless. :)

4. Method of enumeration of options

What if all these tricks don't work? If the inequality does not reduce to non-negative tails, if it is impossible to isolate the module, if at all pain-sadness-longing?

Then the “heavy artillery” of all mathematics enters the scene - the enumeration method. With regard to inequalities with the modulus, it looks like this:

1. Write out all submodule expressions and equate them to zero;
2. Solve the resulting equations and mark the found roots on one number line;
3. The straight line will be divided into several sections, within which each module has a fixed sign and therefore unambiguously expands;
4. Solve the inequality on each such section (you can separately consider the boundary roots obtained in paragraph 2 - for reliability). Combine the results - this will be the answer. :)

Well, how? Weak? Easily! Only for a long time. Let's see in practice:

Task. Solve the inequality:

\[\left| x+2 \right| \lt\left| x-1 \right|+x-\frac(3)(2)\]

Decision. This crap doesn't boil down to inequalities like $\left| f\right| \lt g$, $\left| f\right| \gt g$ or $\left| f\right| \lt\left| g \right|$, so let's go ahead.

We write out submodule expressions, equate them to zero and find the roots:

\[\begin(align) & x+2=0\Rightarrow x=-2; \\ & x-1=0\Rightarrow x=1. \\\end(align)\]

In total, we have two roots that divide the number line into three sections, inside which each module is revealed uniquely:

Splitting the number line by zeros of submodular functions

Let's consider each section separately.

1. Let $x \lt -2$. Then both submodule expressions are negative, and the original inequality is rewritten as follows:

\[\begin(align) & -\left(x+2 \right) \lt -\left(x-1 \right)+x-1,5 \\ & -x-2 \lt -x+1+ x-1.5 \\ & x \gt 1.5 \\\end(align)\]

We got a fairly simple constraint. Let's intersect it with the original assumption that $x \lt -2$:

\[\left\( \begin(align) & x \lt -2 \\ & x \gt 1,5 \\\end(align) \right.\Rightarrow x\in \varnothing \]

Obviously, the variable $x$ cannot simultaneously be less than −2 but greater than 1.5. There are no solutions in this area.

1.1. Let's separately consider the boundary case: $x=-2$. Let's just substitute this number into the original inequality and check: does it hold?

\[\begin(align) & ((\left. \left| x+2 \right| \lt \left| x-1 \right|+x-1,5 \right|)_(x=-2) ) \\ & 0 \lt \left| -3 \right|-2-1.5; \\ & 0 \lt 3-3.5; \\ & 0 \lt -0,5\Rightarrow \varnothing . \\\end(align)\]

Obviously, the chain of calculations has led us to the wrong inequality. Therefore, the original inequality is also false, and $x=-2$ is not included in the answer.

2. Now let $-2 \lt x \lt 1$. The left module will already open with a "plus", but the right one is still with a "minus". We have:

\[\begin(align) & x+2 \lt -\left(x-1 \right)+x-1.5 \\ & x+2 \lt -x+1+x-1.5 \\& x \lt -2.5 \\\end(align)\]

Again we intersect with the original requirement:

\[\left\( \begin(align) & x \lt -2,5 \\ & -2 \lt x \lt 1 \\\end(align) \right.\Rightarrow x\in \varnothing \]

And again, the empty set of solutions, since there are no numbers that are both less than −2.5 and greater than −2.

2.1. And again a special case: $x=1$. We substitute into the original inequality:

\[\begin(align) & ((\left. \left| x+2 \right| \lt \left| x-1 \right|+x-1,5 \right|)_(x=1)) \\ & \left| 3\right| \lt\left| 0 \right|+1-1.5; \\ & 3 \lt -0.5; \\ & 3 \lt -0,5\Rightarrow \varnothing . \\\end(align)\]

Similarly to the previous "special case", the number $x=1$ is clearly not included in the answer.

3. The last piece of the line: $x \gt 1$. Here all modules are expanded with a plus sign:

\[\begin(align) & x+2 \lt x-1+x-1.5 \\ & x+2 \lt x-1+x-1.5 \\ & x \gt 4.5 \\ \end(align)\]

And again we intersect the found set with the original constraint:

\[\left\( \begin(align) & x \gt 4,5 \\ & x \gt 1 \\\end(align) \right.\Rightarrow x\in \left(4,5;+\infty \right)\]

Finally! We have found the interval, which will be the answer.

Answer: $x\in \left(4,5;+\infty \right)$

Finally, one note that may save you from stupid mistakes when solving real problems:

Solutions of inequalities with modules are usually continuous sets on the number line - intervals and segments. Isolated points are much rarer. And even more rarely, it happens that the boundaries of the solution (the end of the segment) coincide with the boundary of the range under consideration.

Therefore, if the boundaries (those very “special cases”) are not included in the answer, then the areas to the left-right of these boundaries will almost certainly not be included in the answer either. And vice versa: the border entered in response, which means that some areas around it will also be responses.

Keep this in mind when you check your solutions.

The more a person understands, the stronger his desire to understand

Thomas Aquinas

The interval method allows you to solve any equations containing the modulus. The essence of this method is to split the numerical axis into several sections (intervals), and it is necessary to split the axis with the zeros of the expressions in the modules. Then, on each of the resulting sections, any submodule expression is either positive or negative. Therefore, each of the modules can be expanded either with a minus sign or with a plus sign. After these actions, it remains only to solve each of the obtained simple equations on the interval under consideration and combine the answers obtained.

Let's consider this method on a specific example.

|x + 1| + |2x – 4| – |x + 3| = 2x - 6.

1) Find the zeros of the expressions in the modules. To do this, we equate them to zero, and solve the resulting equations.

x + 1 = 0 2x – 4 = 0 x + 3 = 0

x = -1 2x = 4 x = -3

2) Arrange the resulting points in the desired order on the coordinate line. They will break the entire axis into four sections.

3) Let's determine on each of the resulting sections the signs of the expressions in the modules. To do this, we substitute in them any numbers from the intervals of interest to us. If the result of the calculation is a positive number, then we put "+" in the table, and if the number is negative, then we put "-". This can be pictured like this:

4) Now we will solve the equation on each of the four intervals, opening the modules with the signs that are in the table. So, consider the first interval:

I interval (-∞; -3). On it, all modules are opened with a "-" sign. We get the following equation:

-(x + 1) - (2x - 4) - (-(x + 3)) \u003d 2x - 6. We present similar terms, having previously opened the brackets in the resulting equation:

X - 1 - 2x + 4 + x + 3 = 2x - 6

The answer received is not included in the considered interval, so it is not necessary to write it in the final answer.

II interval [-3; -one). At this interval in the table there are signs "-", "-", "+". This is how we reveal the modules of the original equation:

-(x + 1) - (2x - 4) - (x + 3) = 2x - 6. Simplify by expanding the brackets:

X - 1 - 2x + 4 - x - 3 \u003d 2x - 6. We present in the resulting equation the following:

x = 6/5. The resulting number does not belong to the interval under consideration, so it is not the root of the original equation.

III interval [-1; 2). We open the modules of the original equation with the signs that are in the figure in the third column. We get:

(x + 1) - (2x - 4) - (x + 3) = 2x - 6. Get rid of the brackets, move the terms containing the variable x to the left side of the equation, and not containing x to the right. Will have:

x + 1 - 2x + 4 - x - 3 = 2x - 6

The number 2 is not included in the considered interval.

IV interval

Example solved.

Example 3 . Solve the inequality 6 X 2 - | X| - 2 ≤ 0

Decision.

Number X can be positive, negative or zero. Therefore, we need to take into account all three circumstances. As you know, they are taken into account in two inequalities: X≥ 0 and X < 0. При X≥ 0, we simply rewrite our original inequality as is, only without the modulo sign:

6x 2 - X - 2 ≤ 0.

Now for the second case: if X < 0. Модулем отрицательного числа является это же число с противоположным знаком. То есть пишем число под модулем с обратным знаком и опять же освобождаемся от знака модуля:

6X 2 - (-X) - 2 ≤ 0.

Expanding the brackets:

6X 2 + X - 2 ≤ 0.

Thus, we have received two systems of equations:

6X 2 - X - 2 ≤ 0
X ≥ 0

6X 2 + X - 2 ≤ 0
X < 0

We need to solve inequalities in systems - which means we need to find the roots of two quadratic equations. To do this, we equate the left-hand sides of the inequalities to zero.

Let's start with the first one:

6X 2 - X - 2 = 0.

How to solve a quadratic equation - see the "Quadric Equation" section. We will immediately name the answer:

X 1 \u003d -1/2, x 2 \u003d 2/3.

From the first system of inequalities, we get that the solution to the original inequality is the entire set of numbers from -1/2 to 2/3. We write the union of solutions for X ≥ 0:
[-1/2; 2/3].

Now let's solve the second quadratic equation:

6X 2 + X - 2 = 0.

Its roots:

X 1 = -2/3, X 2 = 1/2.

Conclusion: when X < 0 корнями исходного неравенства являются также все числа от -2/3 до 1/2.

Let's combine the two answers and get the final answer: the solution is the whole set of numbers from -2/3 to 2/3, including these extreme numbers.

Answer: -2/3 ≤ X ≤ 2/3.

Or: X ∈ [-2/3; 2/3].

CONSIDERED

Pedagogical Council MOU

"Zashizhma secondary school"

Protocol #1

AGREED

Deputy Director for UVR

_______ /Sidorkina R.L./

APPROVE

Head teacher:

A.P. Konakov

Order No. 63

Solving Equations and Inequalities with Modulus

Research

The program was made up of:

higher mathematics teacher

Sidorkina R.L.

v. Zashizhemye, 2014

Table of contents

Introduction……………………………………………………………………3

The simplest equations and inequalities with the modulus……………………5

Graphical solution of equations and inequalities with the module………….8

Other ways to solve equations and inequalities with the modulus……......10

Conclusion ……………………………………………………………..16

References……………………………………………………………18

1. Introduction

The concept of an absolute value (modulus) is one of the most important characteristics of a number both in the field of real and in the field of complex numbers.

This concept is widely used not only in various sections of the school mathematics course, but also in the courses of higher mathematics, physics and technical sciences studied at universities. For example, in the theory of approximate calculations, the concepts of absolute and relative errors of an approximate number are used. In mechanics and geometry, the concepts of a vector and its length (vector modulus) are studied. In mathematical analysis, the concept of the absolute value of a number is contained in the definitions of such basic concepts as a limit, a bounded function, etc. Problems related to absolute values ​​are often found at mathematical Olympiads, entrance exams to universities and the Unified State Examination. And so it became important for us to study some aspects of this topic.

Home purpose in our work is the study of various methods for solving equations and inequalities with modules.

This goal should be achieved by solving the following tasks:

Examine the definition and some properties of the module.

Master the solution of the simplest equations and inequalities with the modulus through equivalent transitions

Consider various methods for solving equations and inequalities with a modulus.

object studies are some types of equations and inequalities with modulus.

Thing research - various methods for solving equations and inequalities with a module, namely: a graphical method, method of geometric interpretation, use of identity, application of the sign theorem, method of transition to a consequence, method of intervals, method of multiplication by a positive factor, method of expansion of modules.

During the study, methods such as the study of the literature on this issue and the practical method were used.

In the course of our work, we researched such sources as:

1. "Big Mathematical Encyclopedia" for schoolchildren and students;

Mathematics. USE - 2011-2012. Standard exam options. / Edited by A.L. Semenova, I.V. Yashchenko.

Encyclopedia "I know the world" Mathematics;

;

1. 1. The simplest equations and inequalities with modulus

We will refer to the simplest equations the equations solved by one of the following equivalent transitions:

Examples of solving the simplest equations.

Example 1 Let's solve the equation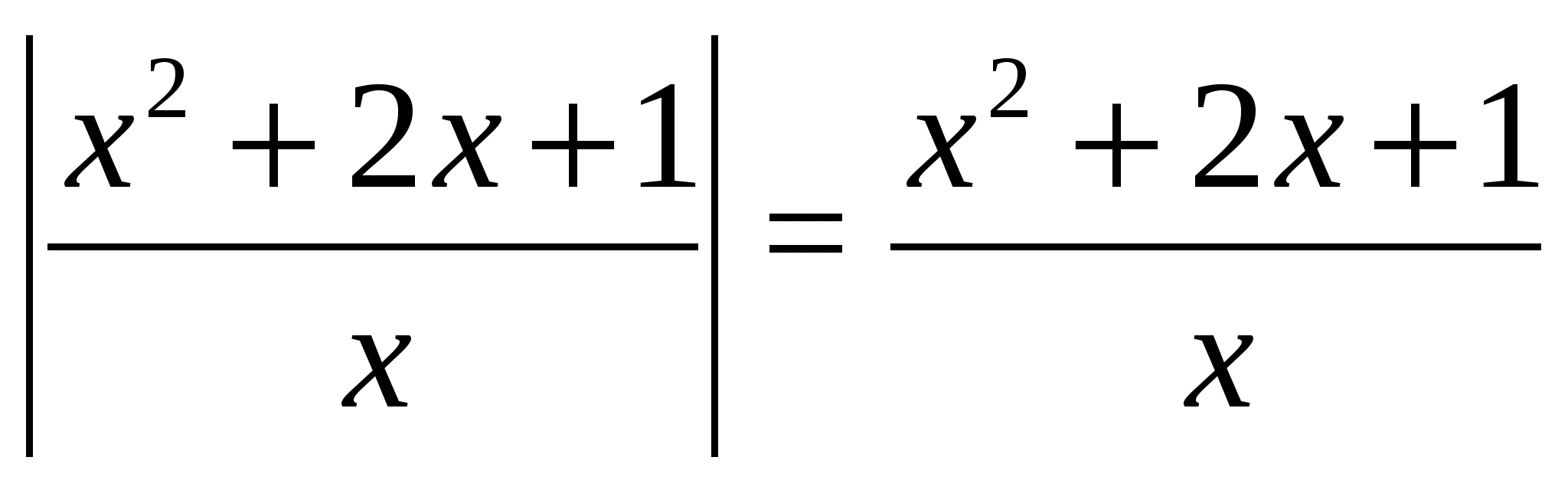
.

Decision.

Answer.
.

Example 2 Let's solve the equation.

Decision.

Answer.
.

Example 3 Let's solve the equation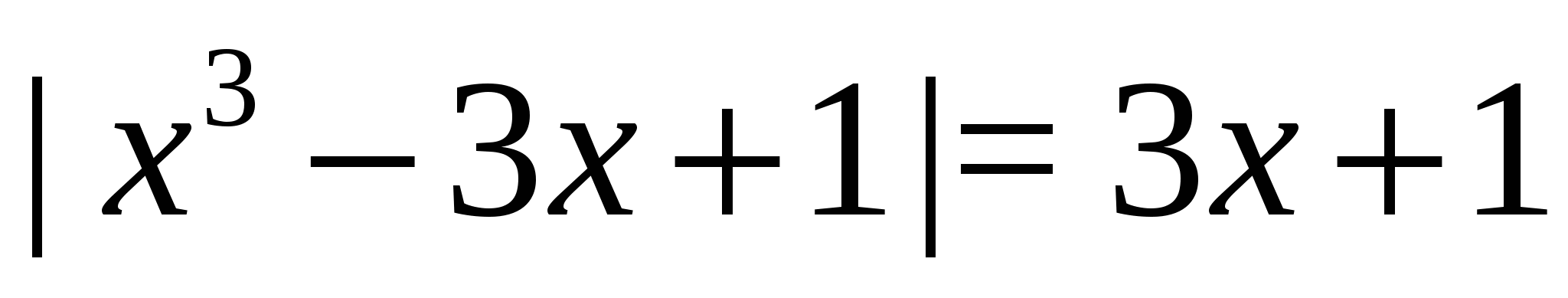
.

Decision.

Answer.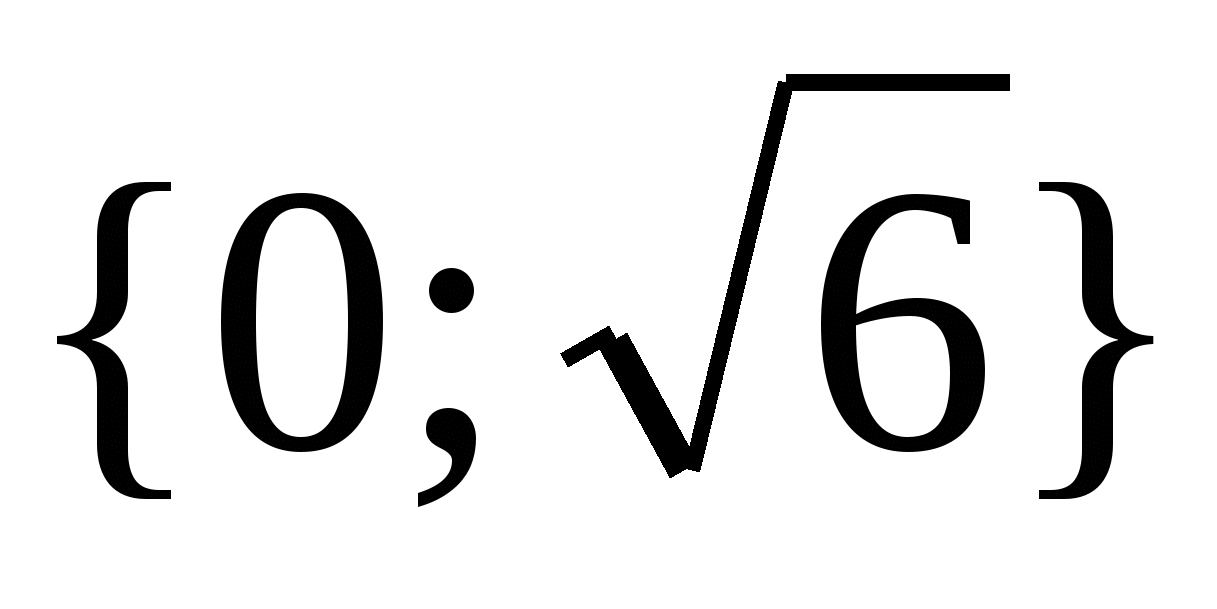
.

A number of equations are solved using the following theorem.

Theorem.4 The sum of modules is equal to the algebraic sum of submodule quantities if and only if each quantity has the sign with which it enters the algebraic sum.

Example 5 solve the equation

Decision. Since , we have an equality of the form , where
,
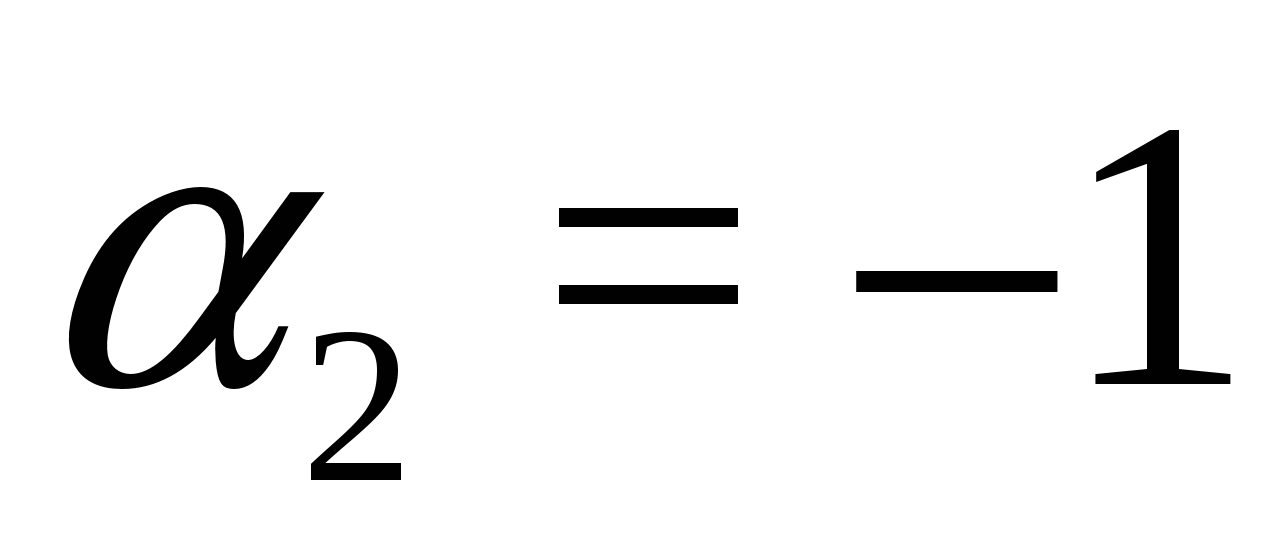. Therefore, the original equation is equivalent to the system:

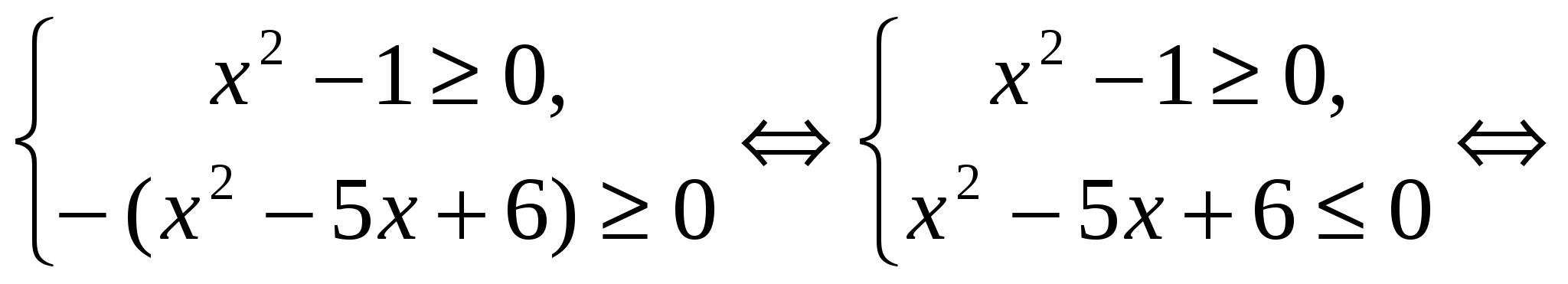
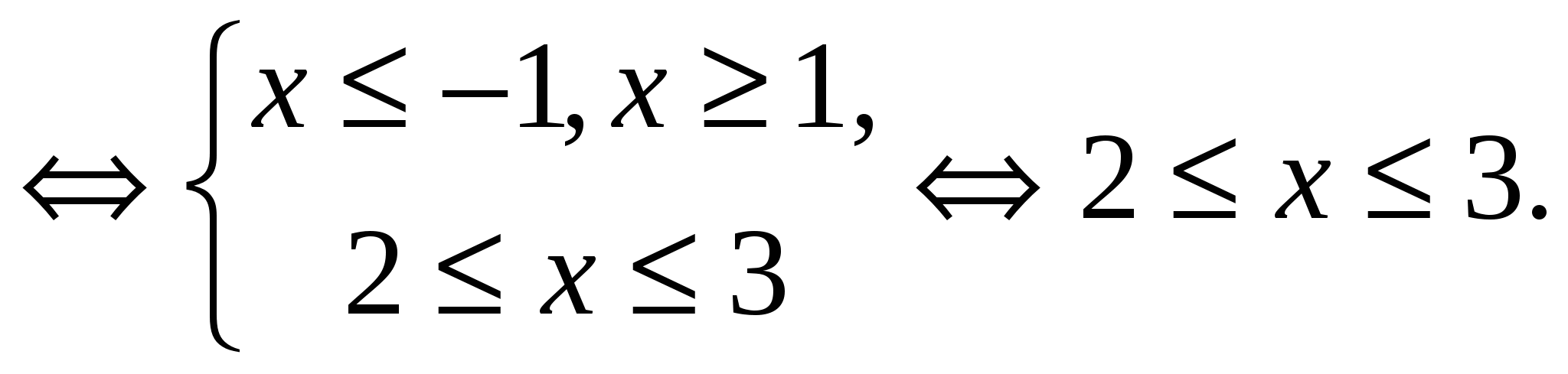
Answer.
.

Examples of solving the simplest inequalities.

Example 6 Let's solve the inequality
.

Decision.

Answer.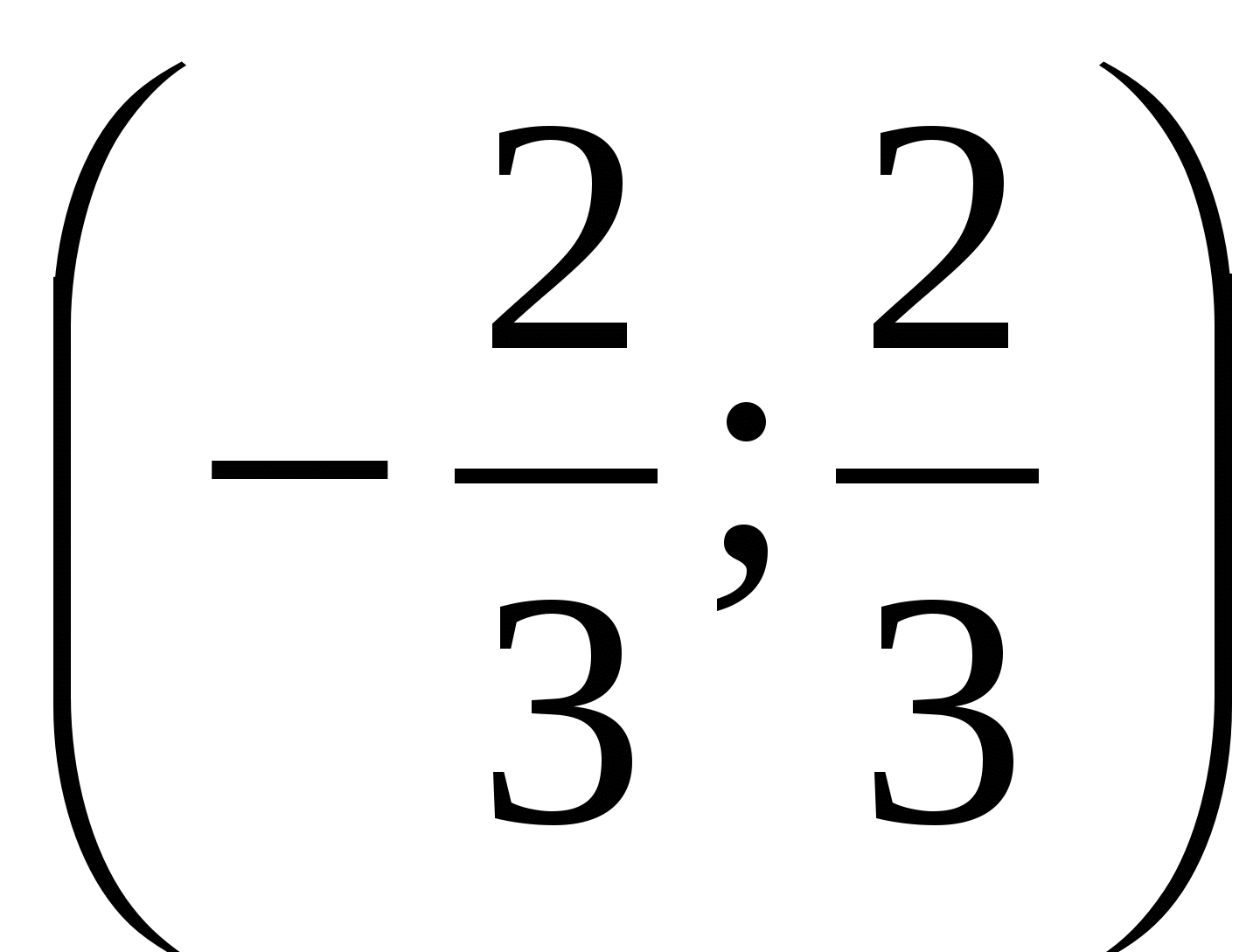
.

Example 7 Let's solve the inequality
.

Decision.

Answer.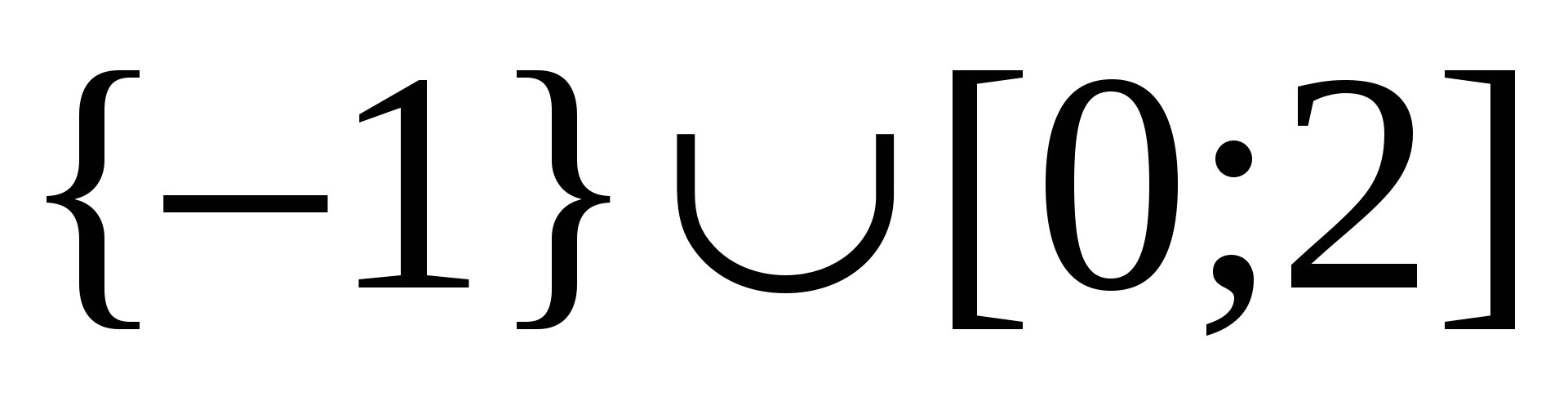
.

Oddly enough, but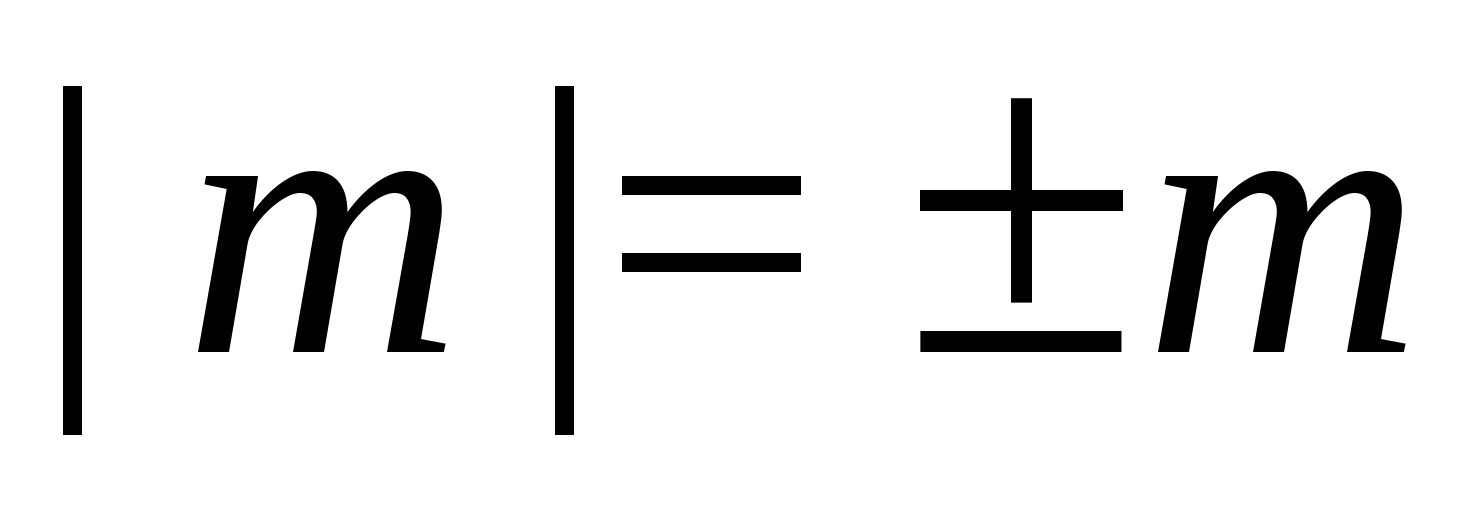
enough to get rid of the modulo sign in any inequalities.

Example 8 Solve the inequality

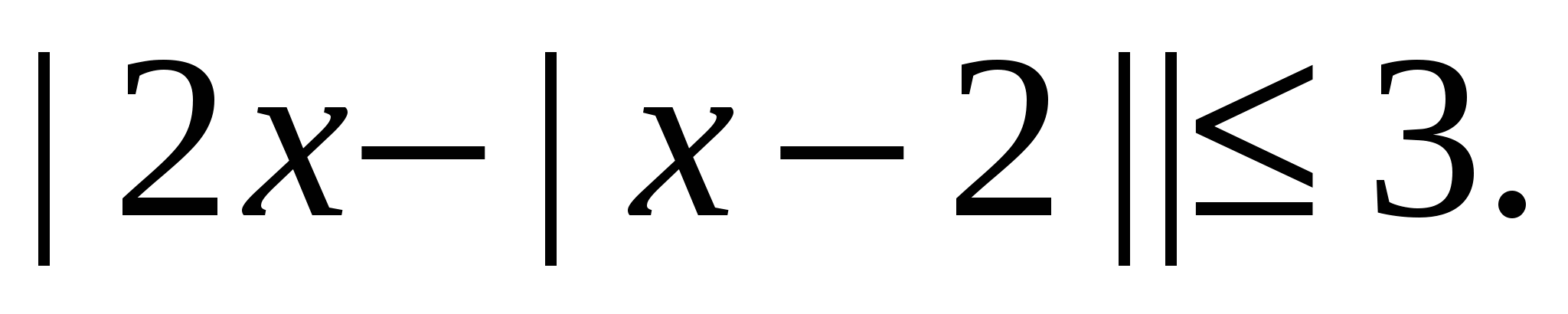
Decision.

Answer.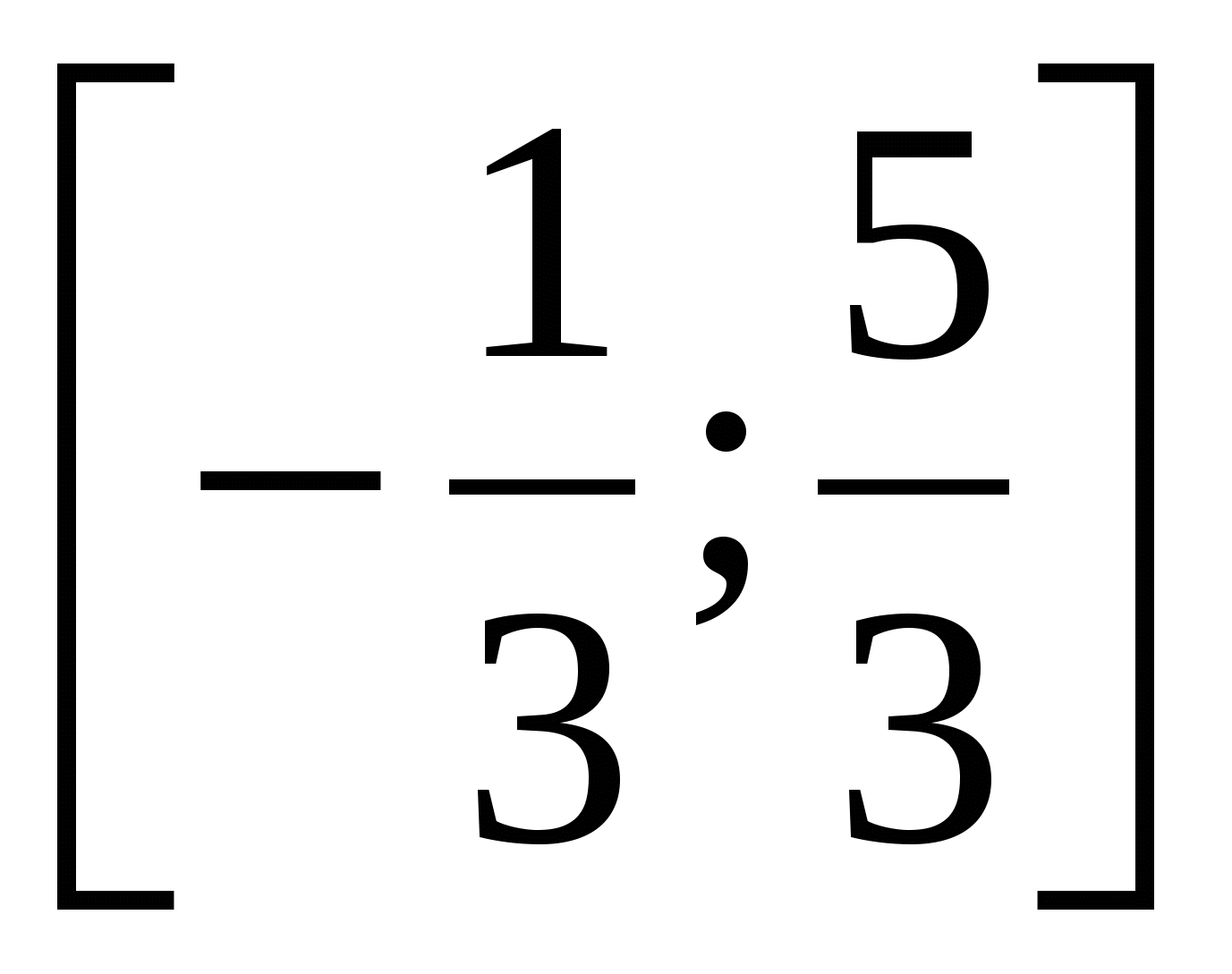
.

3. Graphical solution of equations and inequalities with modulus

The solution of equations containing the sign of the absolute value is often much more convenient to solve not analytically, but graphically (especially equations containing parameters).

Example 9(C5, USE - 2010)

C5. For each valuea indicate the number of solutions to the equation

Decision.Let's plot the function
. To do this, select a full square:

The number of points of intersection of the graph of the function y \u003d
with horizontal lines y = a is equal to the number of solutions to the equation.

O reply: if < 0, то решений нет; если а= 0, то два решения, если 0 < а < 4, то четыре решения; если а=4, то три решения; если а >4, then two solutions.

Other ways to solve equations and inequalities with the modulus

• Module expansion method

Let's look at the module expansion method using an example:

Example 10 solve the equation

Decision. This equation contains more than one module.

The method for solving equations containing variables under the sign of two or more modules is as follows.

1. Find the values ​​of the variable for which each of the modules vanishes: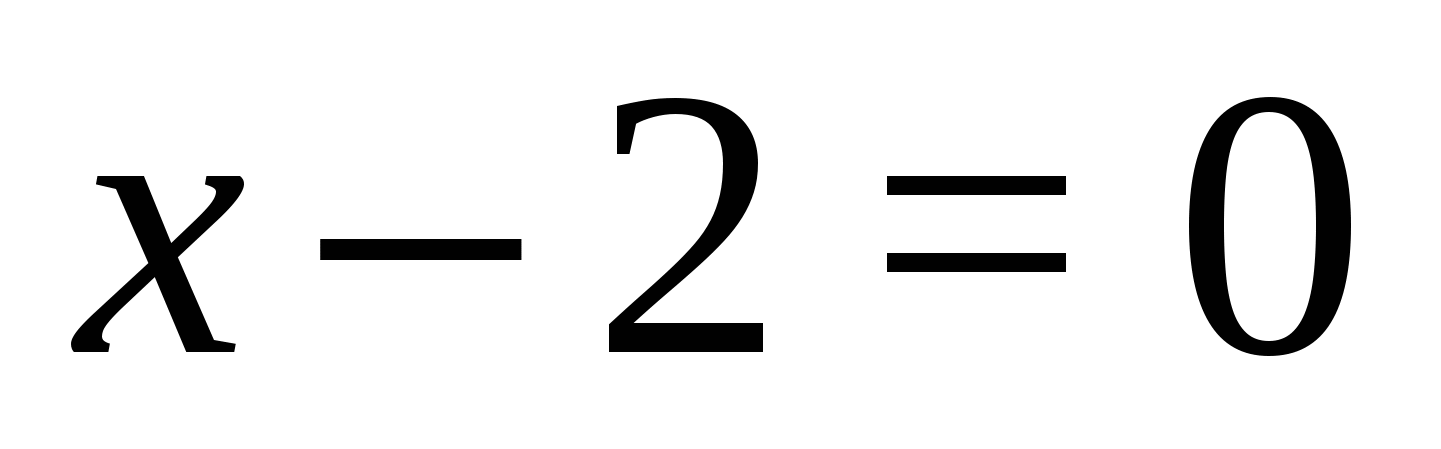
,
;
,
;
,
.

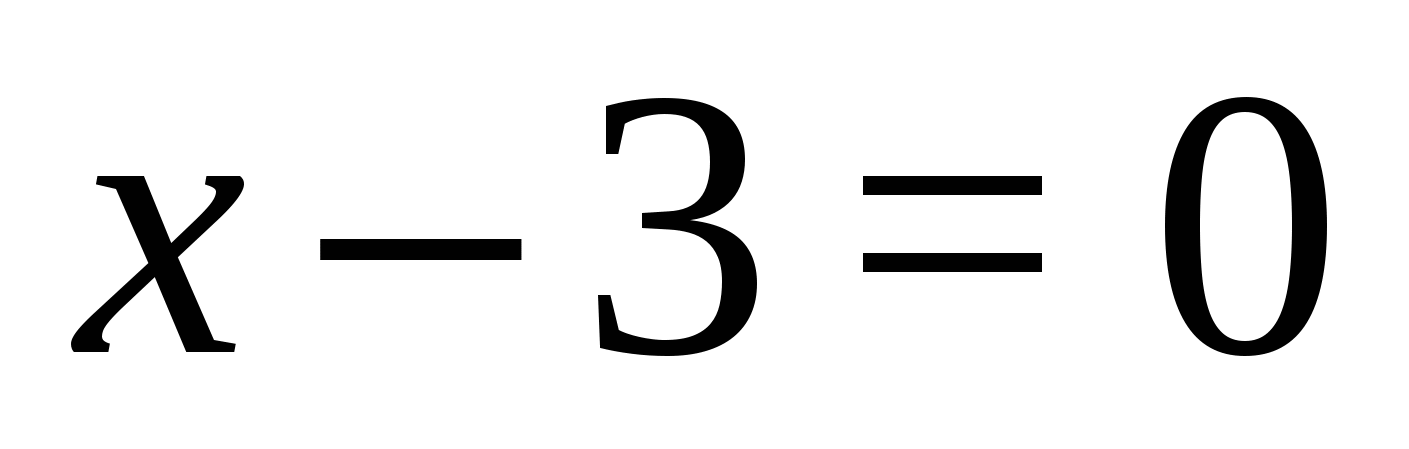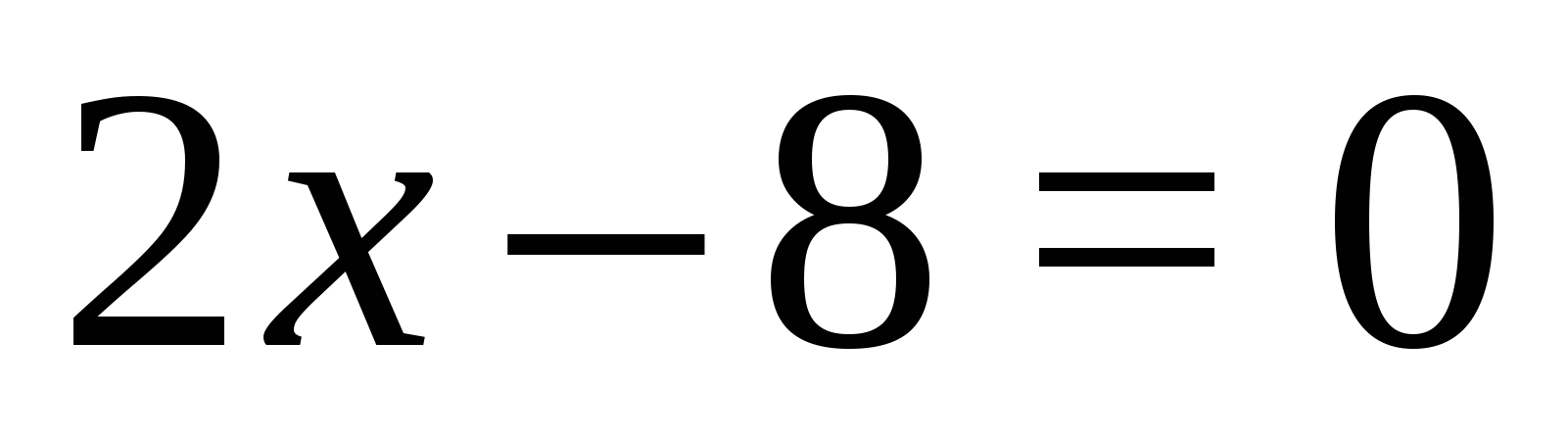2. Mark these points on the number line.

3. We consider the equation on each of the intervals and set the sign of the expressions that are under the modules.

1) When
or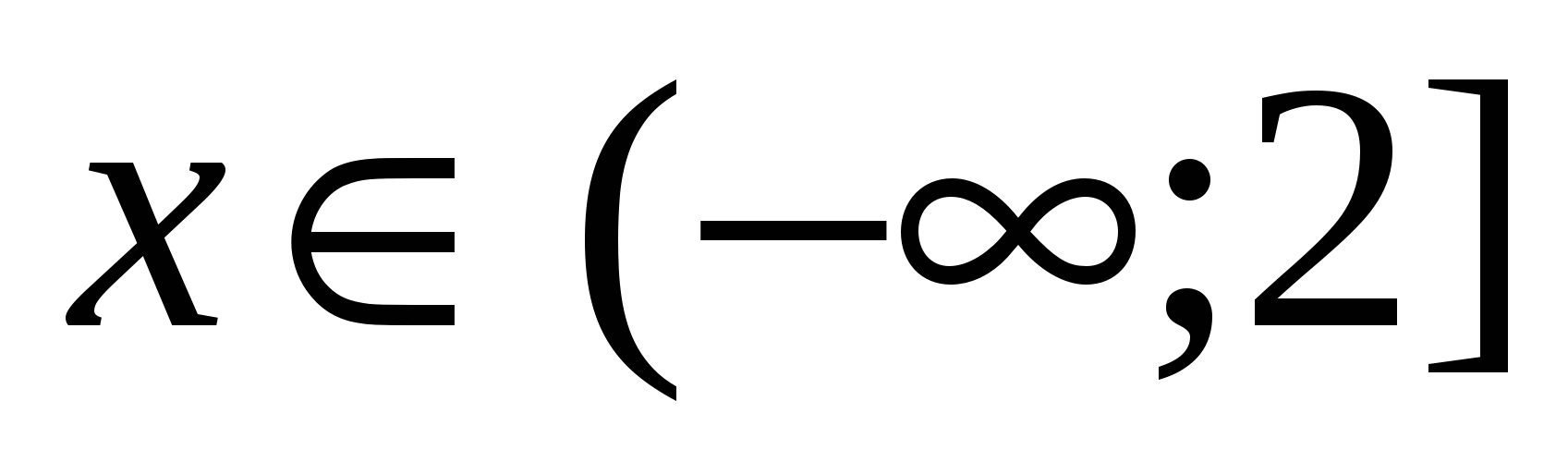
. To determine the sign of each of the expressions under the modulus on this interval, it is enough to take any value 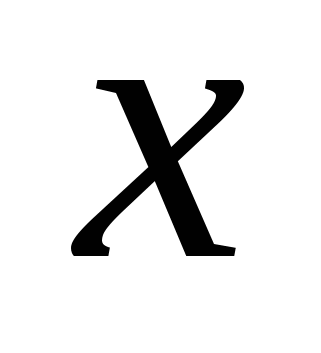from this interval and substitute into the expression. If the value obtained is negative, then for all 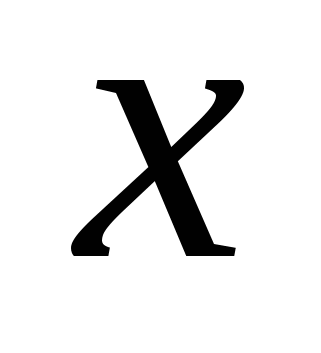from this interval the expression will be negative; if the resulting numerical value is positive, then for all values 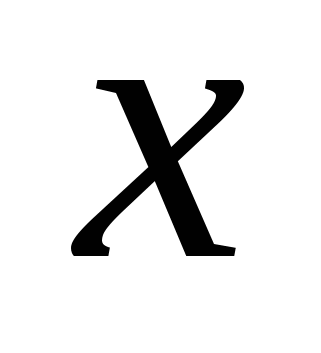from this interval the expression will be positive.

Let's take the value
from the gap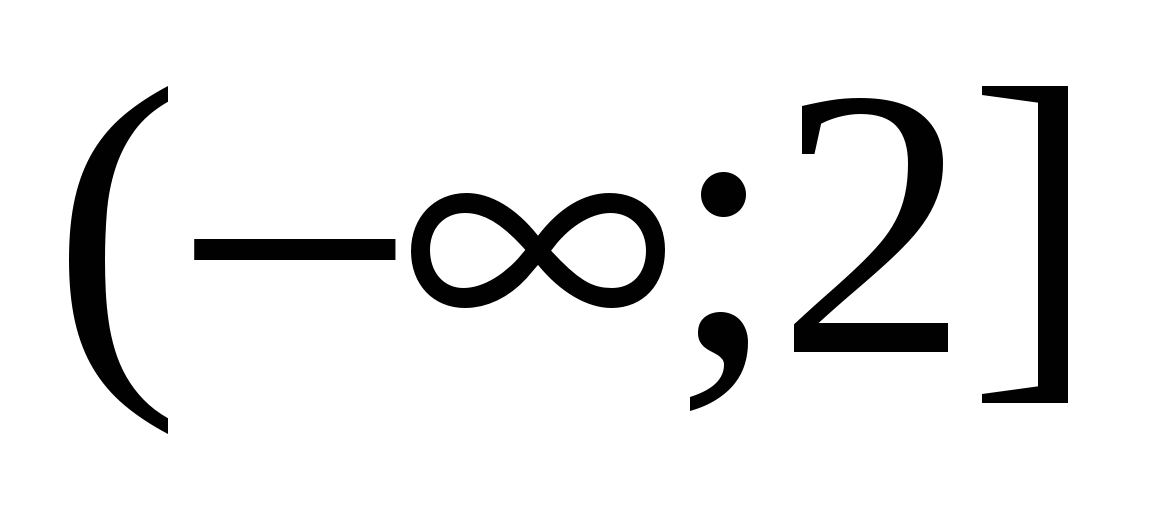
and substitute its value into the expression
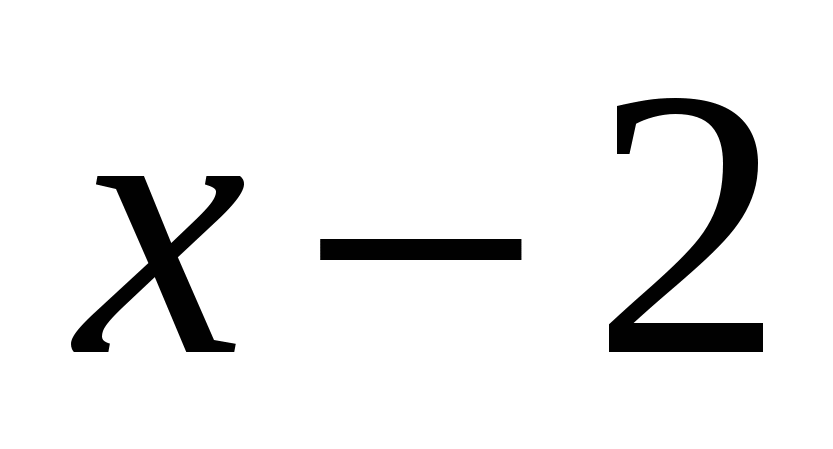, we get
, so on this interval
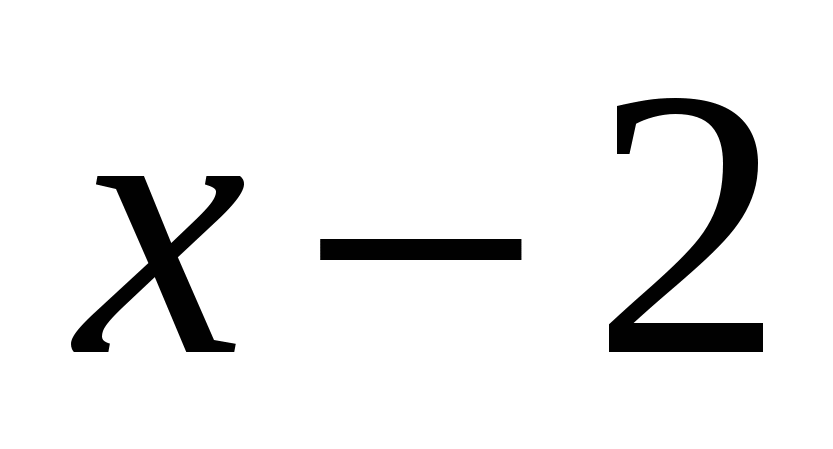is negative, and therefore ``out" from under the module with a ``minus sign"", we get:
.

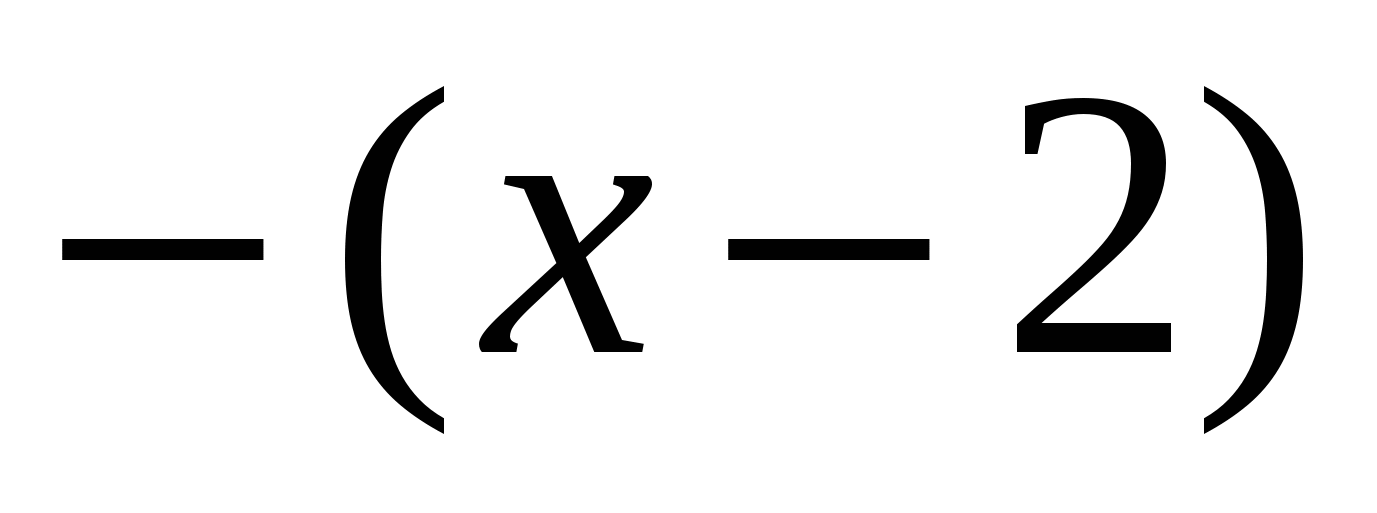With this value , expression
will get the value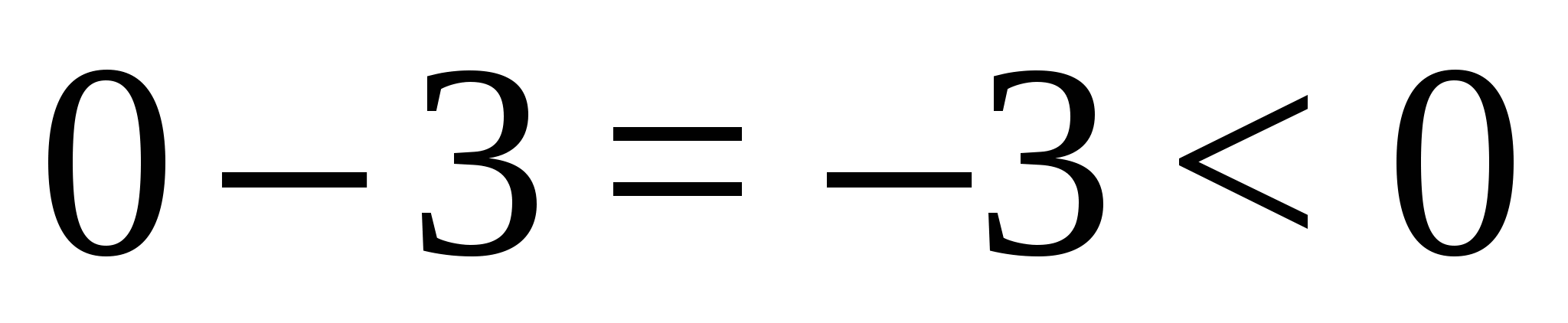
, so it is in the interval
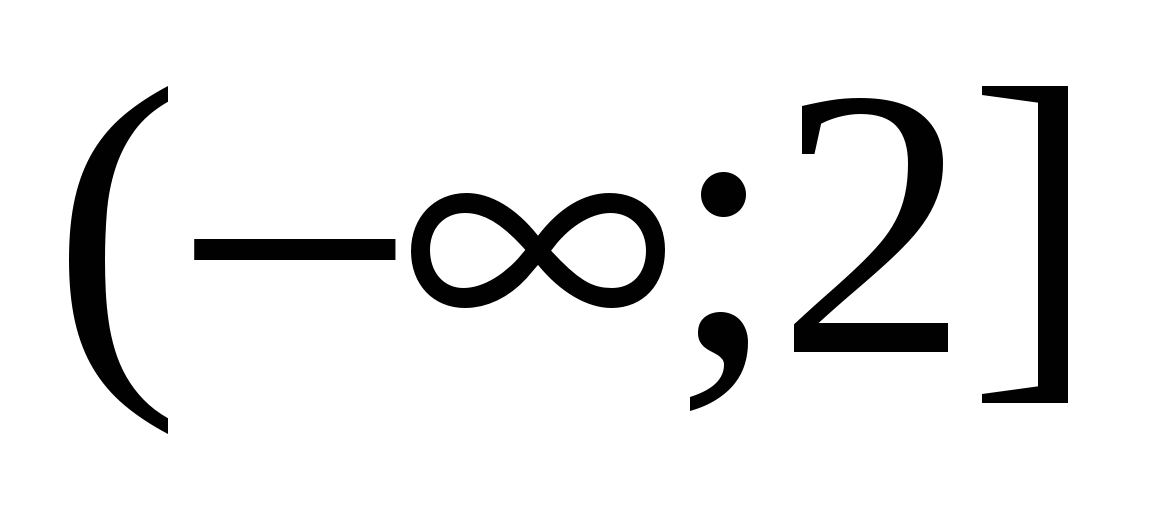also accepts negative values ​​and ``leaves"" from the module with a ``minus"' sign, we get:
.

Expression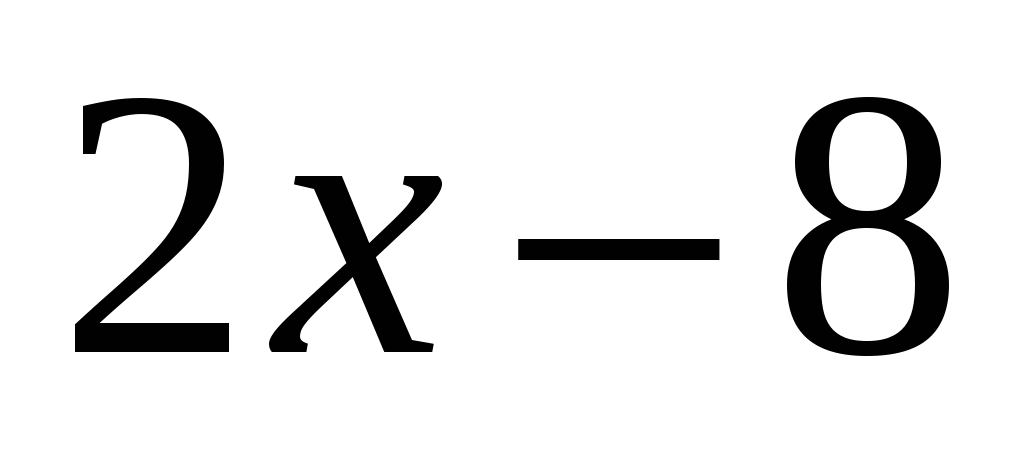
will get the value
and "exits" the module with a ``minus"" sign:
.

The equation on this interval will turn out as follows: , solving it, we find:
.

Find out if this value is in the range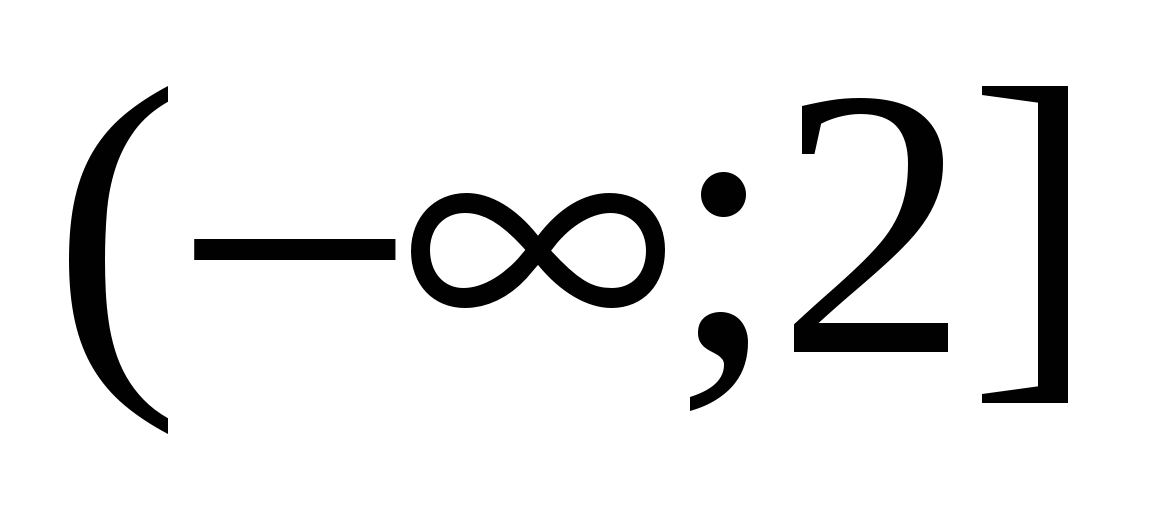
. Turns out it's included
is the root of the equation.

2) When
. Choose any value 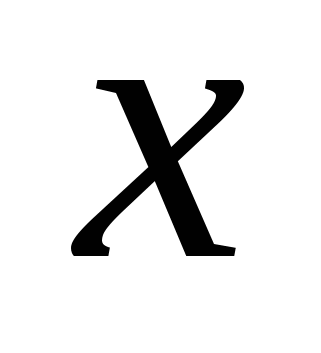from this interval. Let be
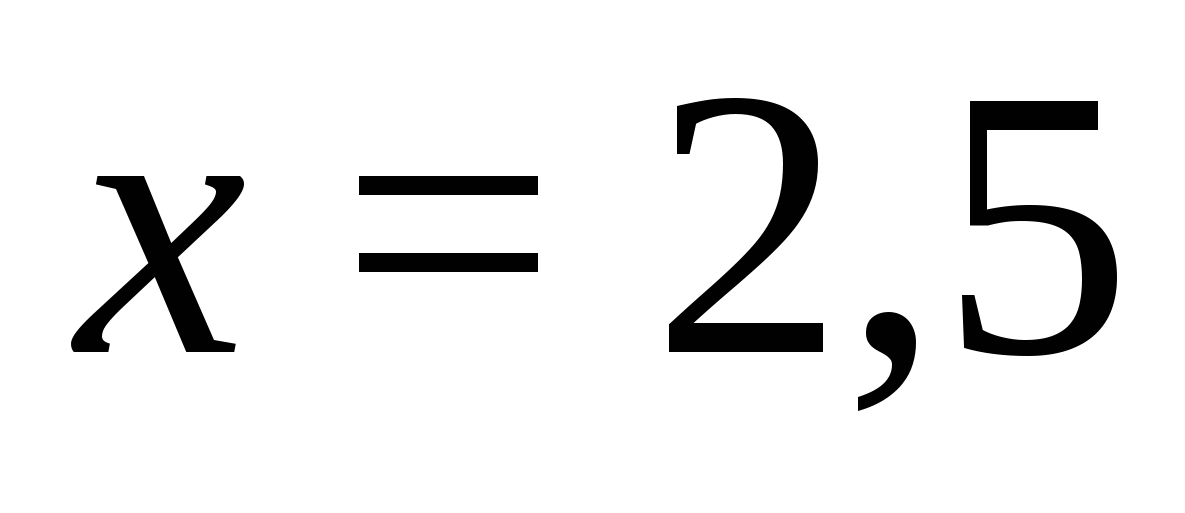. We determine the sign of each of the expressions under the module at this value 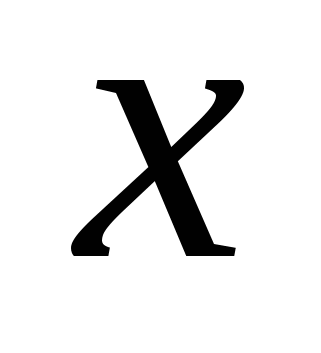. It turns out that the expression
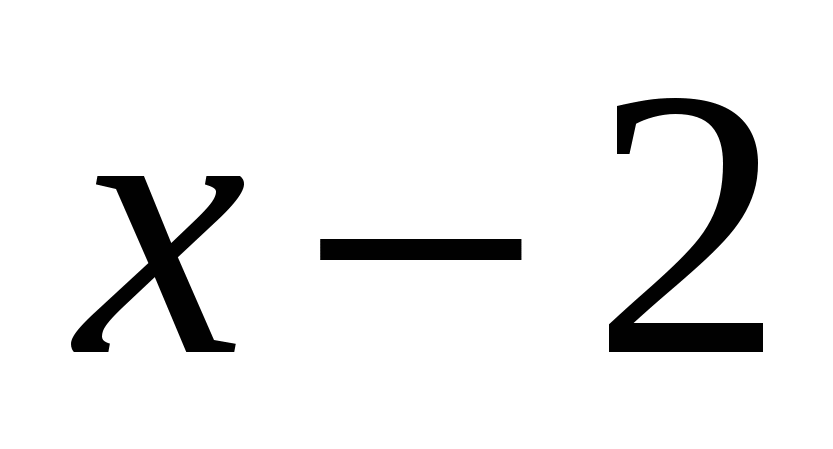positive and the other two are negative.

The equation on this interval will take the form: . Solving it, we find
. This value is not included in the range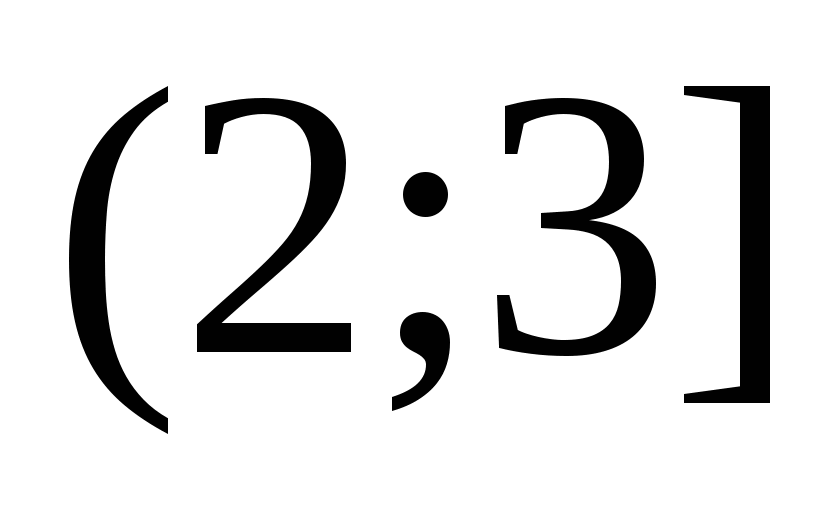
, which means it is not a root of the equation.

3) When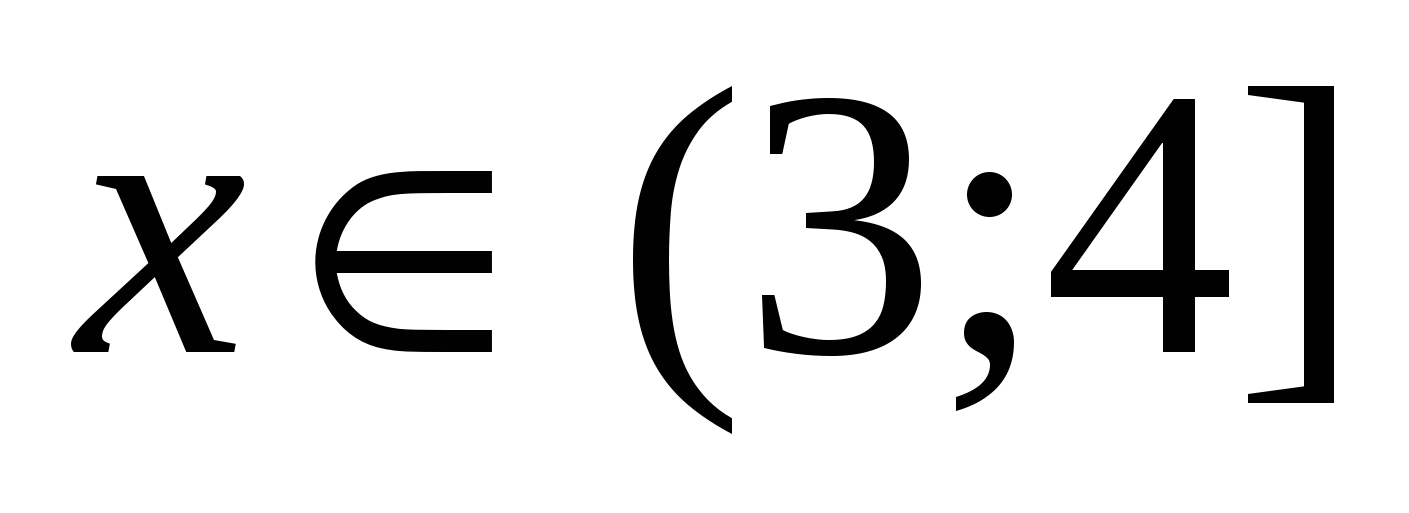
. Choose an arbitrary value 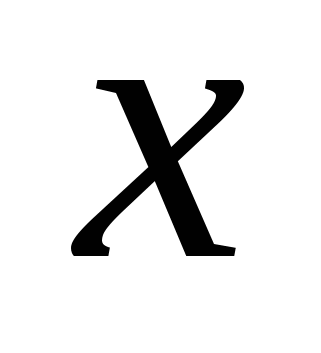from this interval, say,
and substitute into each of the expressions. We find that the expressions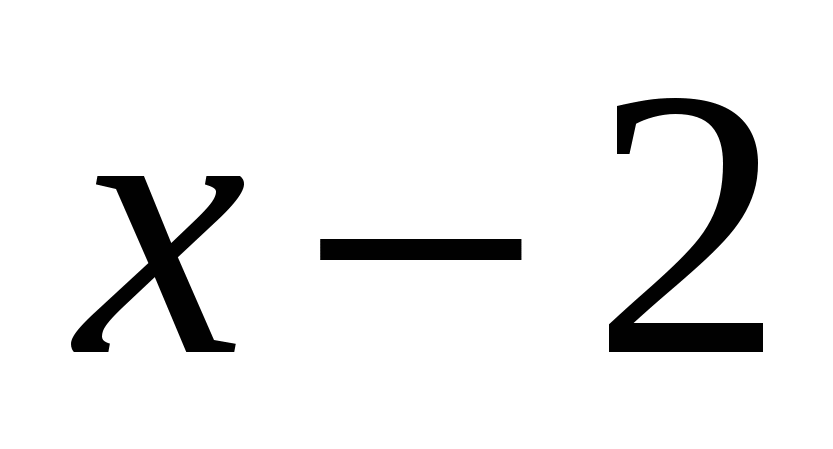
and
are positive and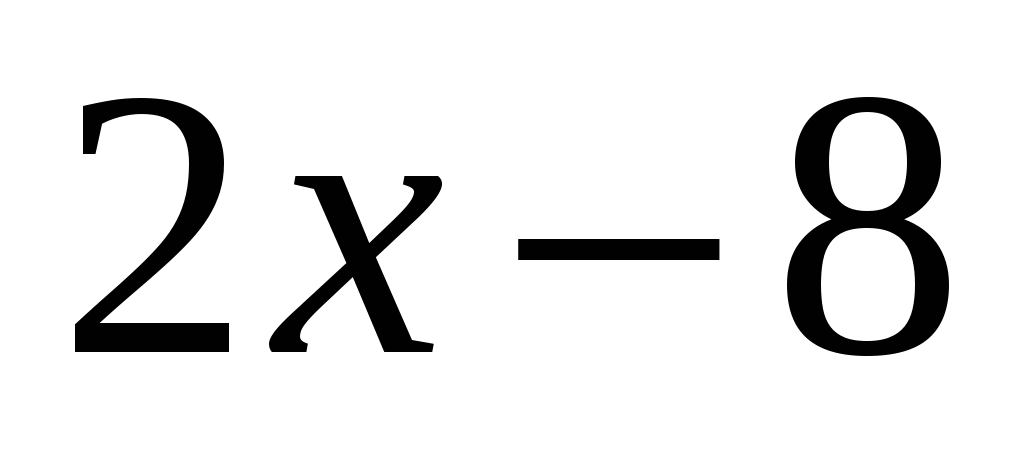
- negatively. We get the following equation: .

After transformation, we get:
, which means that the equation has no roots on this interval.

4) When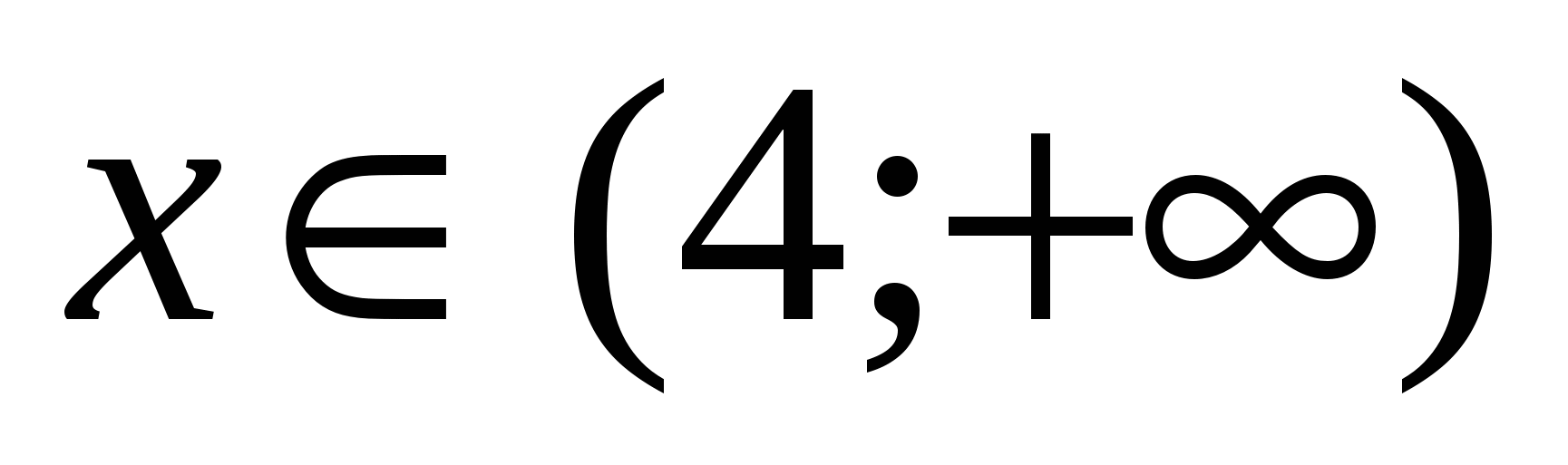
. It is easy to establish that all expressions on this interval are positive, which means we get the equation:
,
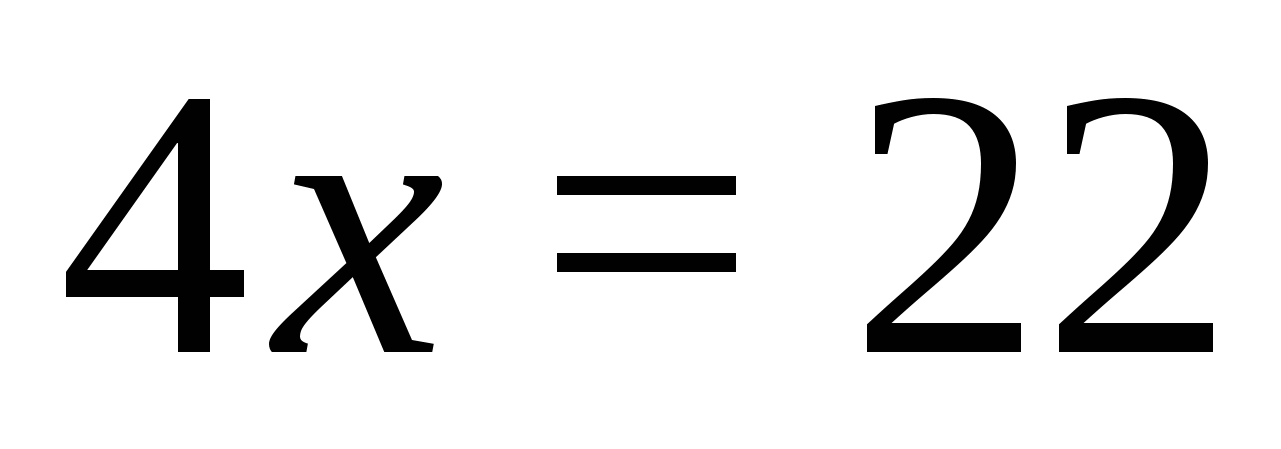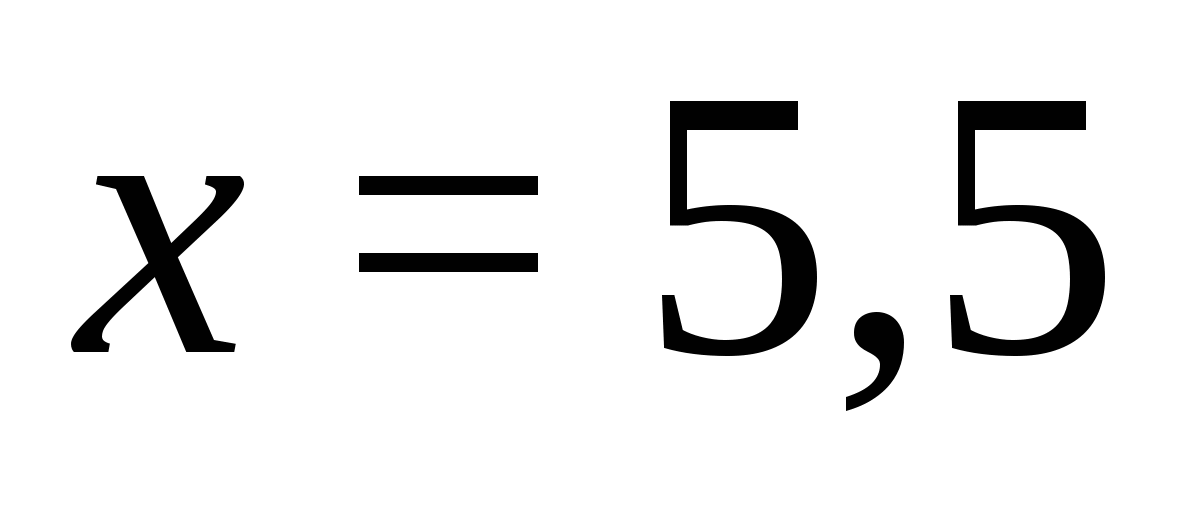which is included in the interval and is the root of the equation.

Answer.
,
.

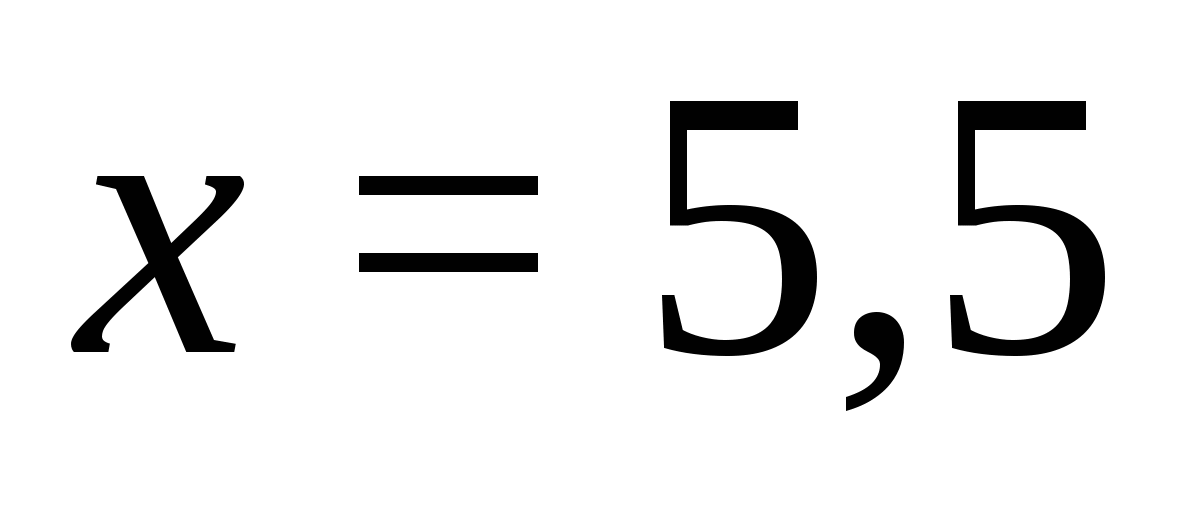• Solution of equations containing moduli of non-negative expressions

Example 11 What is the sum of the roots of the equation (the root, if it is one) of the equation

Decision. Consider the expression

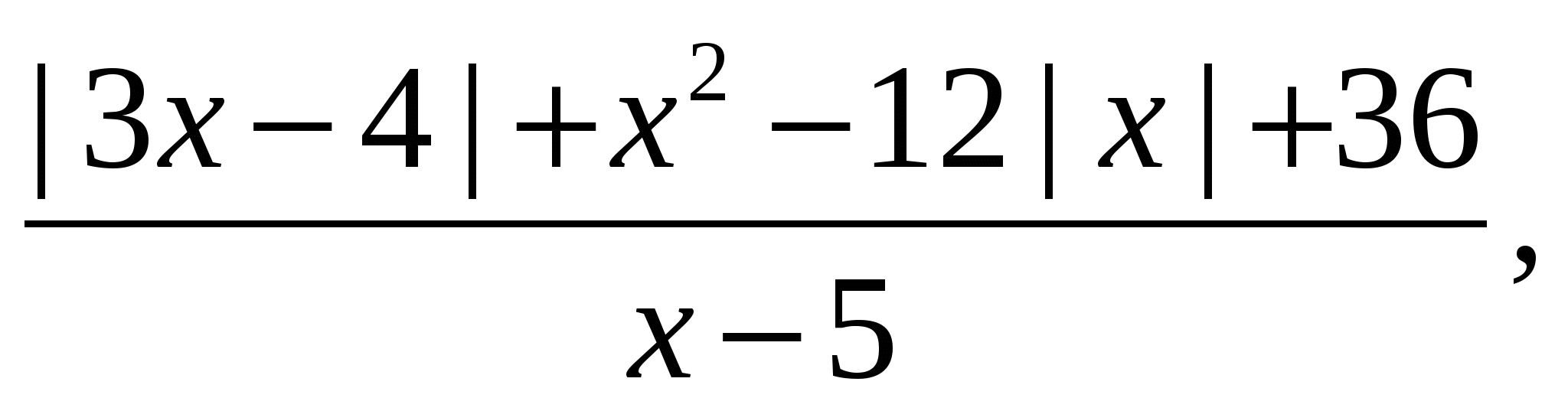and transform it to the form

It is obvious that the numerator of a fraction for any values ​​of the variable is a positive number. So the fractional expression is positive if
(because
). We transform the resulting expression, provided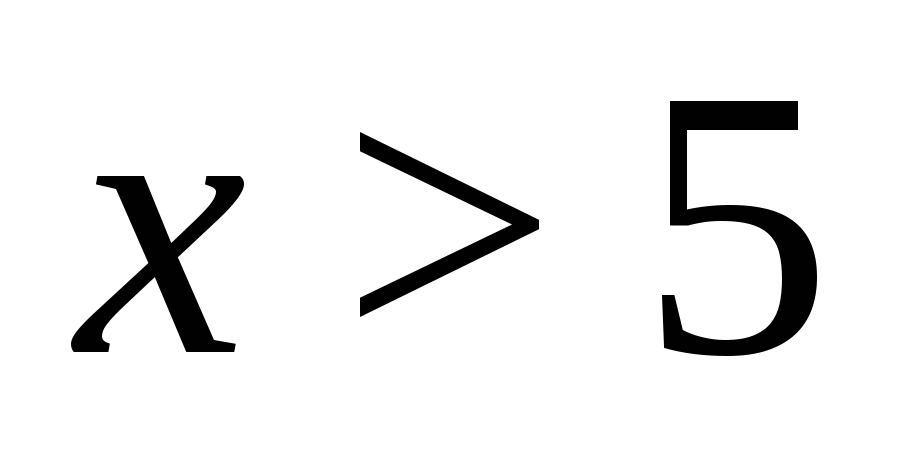
. We get an equation equivalent to the original one:

Answer.
.

Example 12 solve the equation

Decision. Since the left side of the equation is non-negative, for all admissible values ​​of the variable, on the set of roots of the equation, its right side must also be non-negative, hence the condition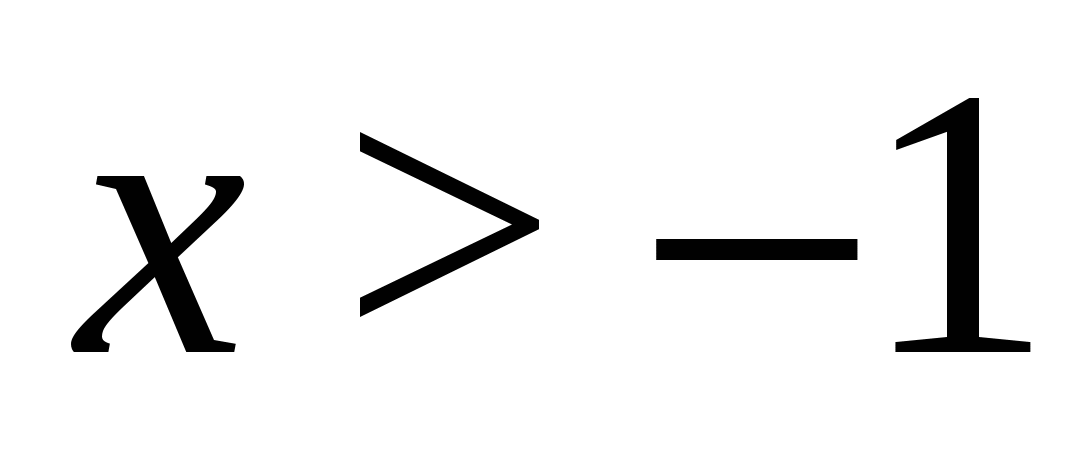
, on this interval the denominators of both fractions are equal, and it remains to solve the equation
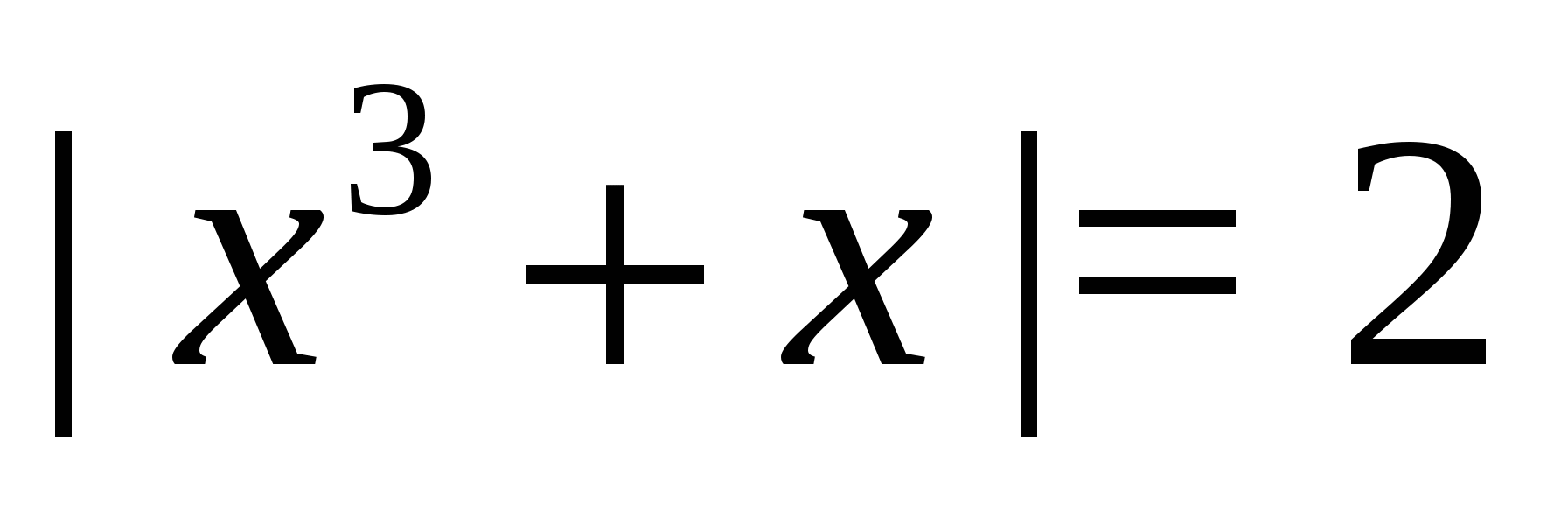. Solving it and considering the constraint
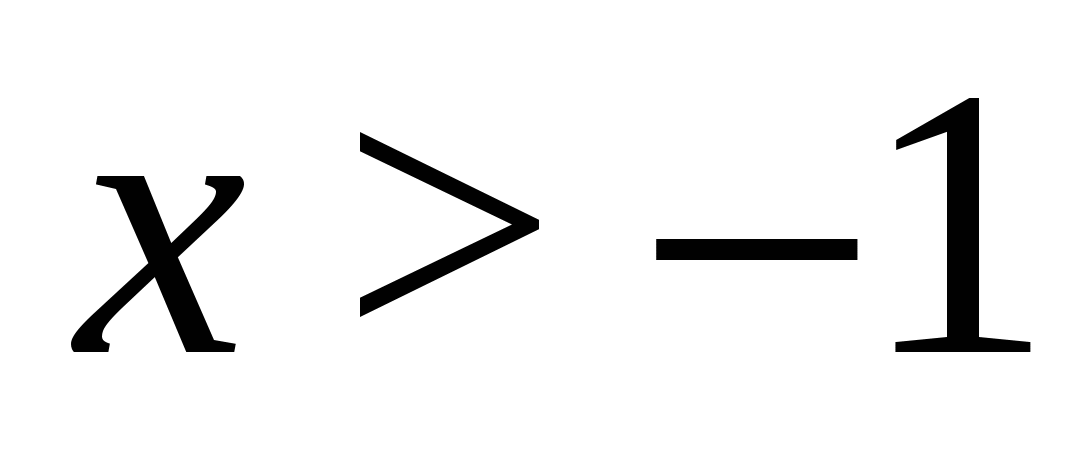, we get

Answer.
.

• Solving Equations Using Geometric Interpretation

The geometric meaning of the expression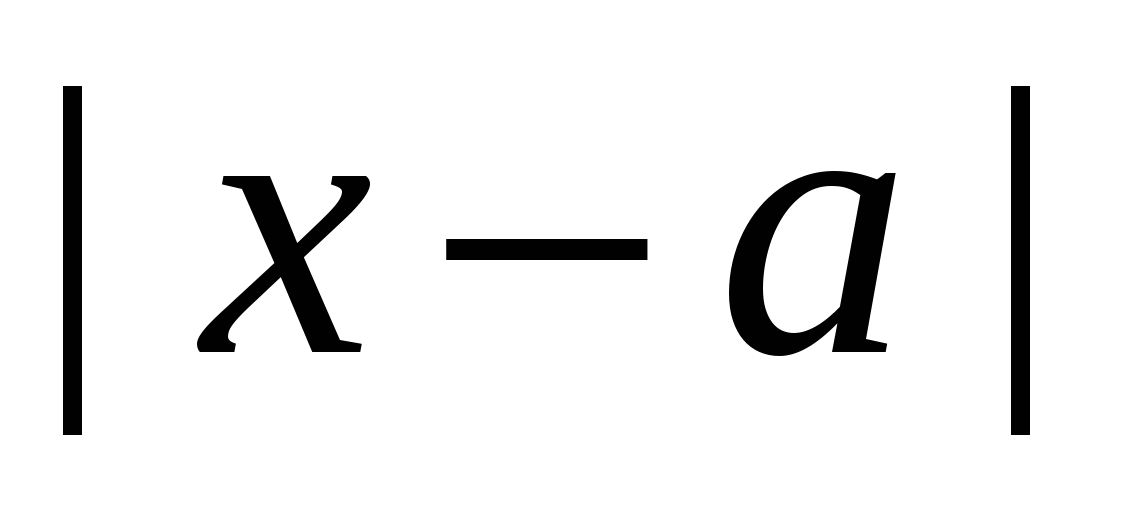
- the length of the segment of the coordinate axis connecting the points with abscissas and . The translation of an algebraic problem into a geometric language often makes it possible to avoid cumbersome calculations.

Example 13 Let's solve the equation
.

Decision. We will argue as follows: based on the geometric interpretation of the modulus, the left side of the equation is the sum of the distances from some point with the abscissa 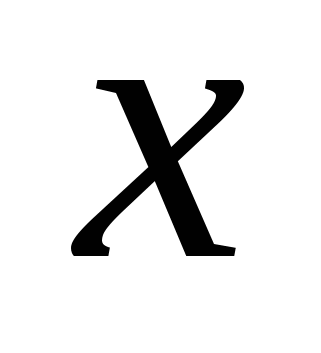up to two fixed points with abscissas 1 and 2. Then all points with abscissas from the segment
have the required property, while points located outside this segment do not.

Answer.
.

Example 14 Solve the inequality
.

Decision. Draw points on the coordinate line, the sum of the distances from which to the points
and is exactly equal to . These are all cut-off points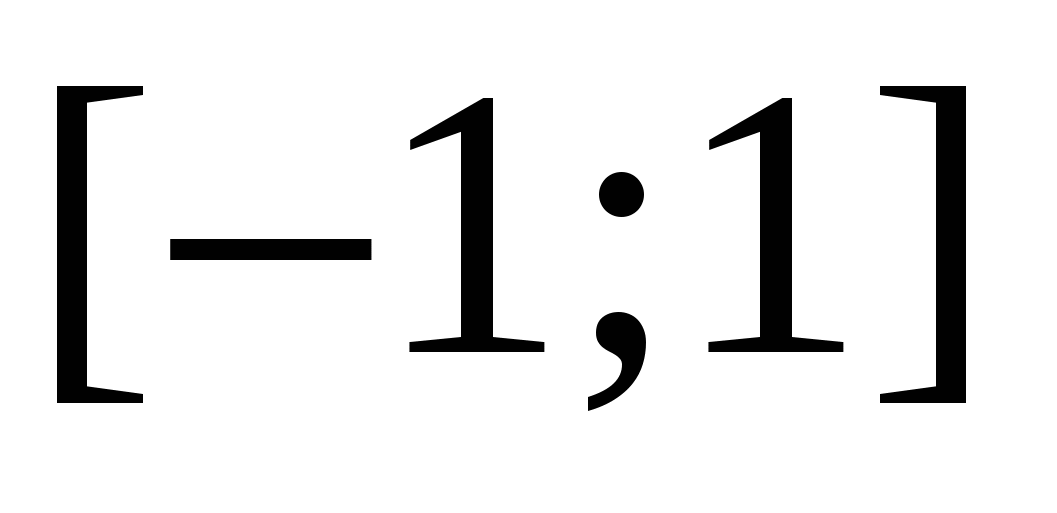
. For all numbers outside this segment, the sum of the distances will be greater than two.

Answer.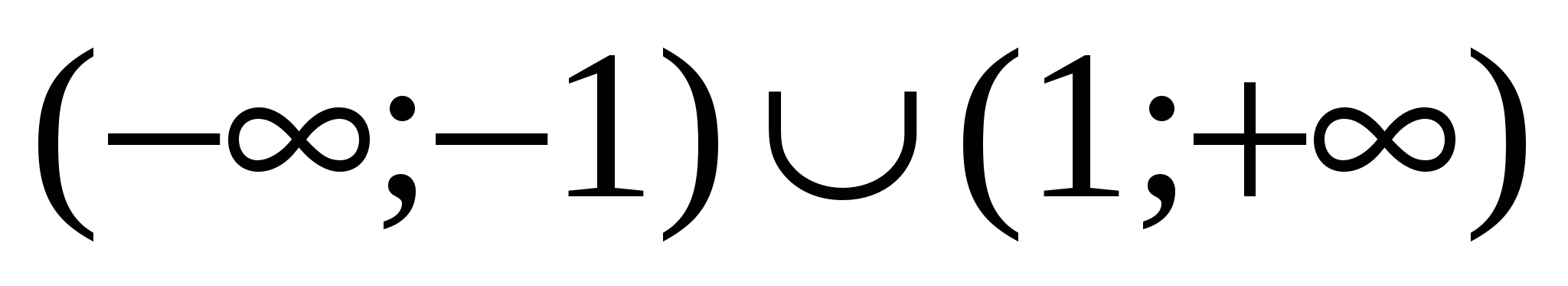
.

Example(C3, USE - 2010) 15 solve the equation

Decision. Applying the identity twice
, we get the equation

whose solution is the interval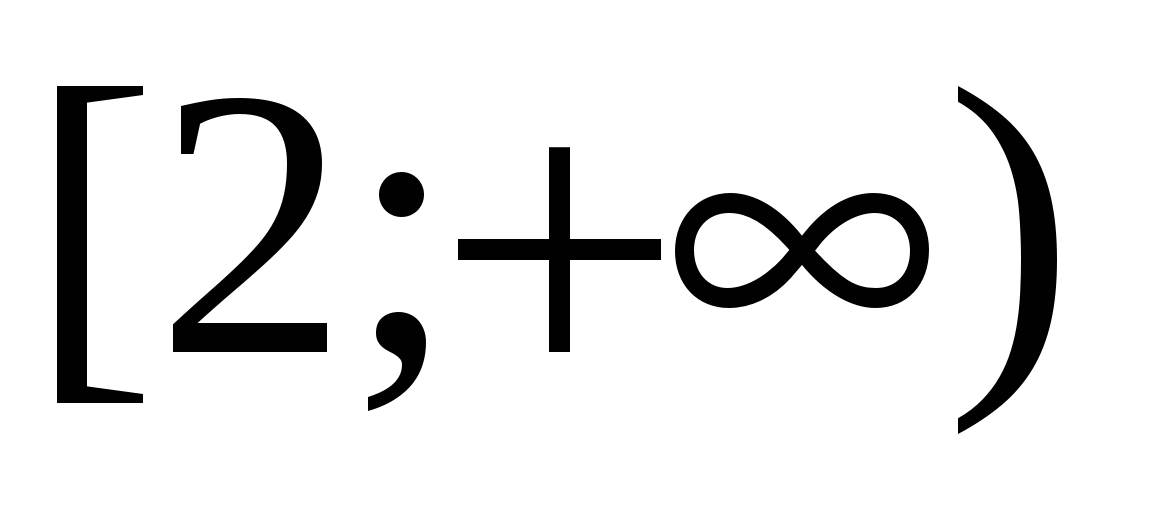
.

Answer.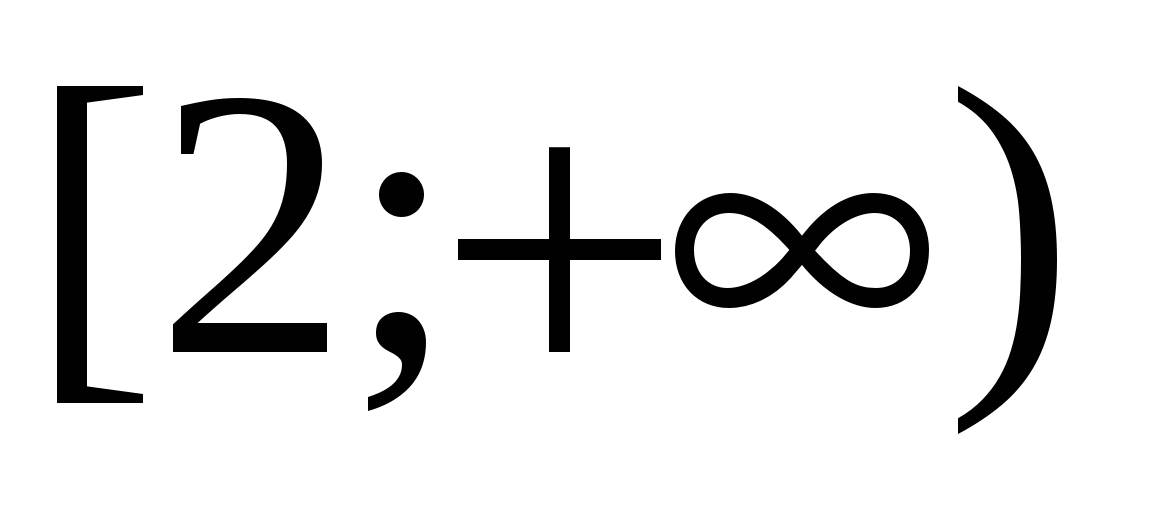
.

Example(C3, USE - 2011) 16 17 solve the equation

Decision. .

Answer.
.

• Applying the sign theorem to solving equations

We formulate a theorem convenient for solving inequalities regarding products or partial differences of moduli:

Theorem 18 The sign of the difference between the modules of two expressions coincides with the sign of the difference of the squares of these expressions. does not vanish for any value of the variable. This means that the function is of constant sign over the entire domain of definition. By calculating, for example,
, we get that the function takes only positive values.

Answer.
.

The interval method allows you to solve more complex equations and inequalities with modules, but in this case it has a slightly different purpose. The point is the following. We find the roots of all submodular expressions and divide the numerical axis into intervals of sign constancy of these expressions. This allows, sequentially going through these intervals, simultaneously getting rid of all the modules and solving the usual equation or inequality (while checking that the found answer is included in the given interval).

• Solving equations by multiplying by a positive factor

Conclusion.

Summing up our work, we can say the following.

The purpose of the work was to study various methods for solving equations and inequalities with modules.

Some varieties of the simplest equations and inequalities with modulus, which can be solved with the help of equivalent transitions, as well as theorems on the sum of moduli are considered; graphical way of solving equations. It must be said that in the school mathematics course, it is these solution methods that are most often used. The graphical method is especially relevant in solving problems C 5 from the control and measuring materials of the exam.

Next, we studied, using several examples, other methods for solving equations and inequalities with modules, namely: the method of expanding modules; solution of equations containing modules of non-negative expressions; solving equations using geometric interpretation; using the identity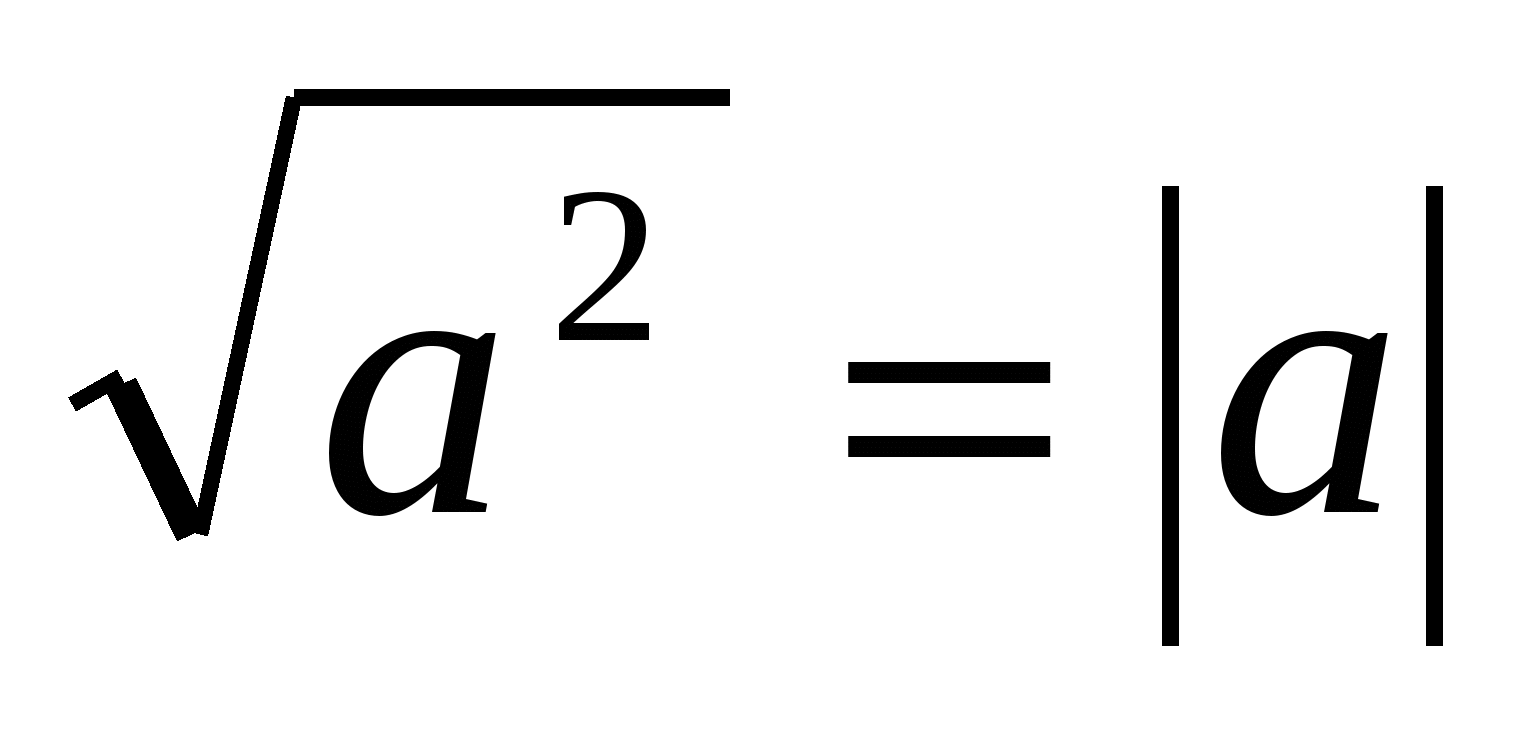
; application of the sign theorem; solving equations by passing to the consequence, multiplying by a positive factor, as well as solving inequalities by the method of intervals.

Thus, in the course of the study, we came to the following conclusions.

We consider the module expansion method, the graphical method and the interval method to be the most universal and applicable to the largest number of tasks. This belief arose as a result of solving a large number of tasks from the test materials of the Unified State Examination, subject championships, Olympiad tasks, as well as studying the literature on this issue. We also consider it very important to know and apply the identity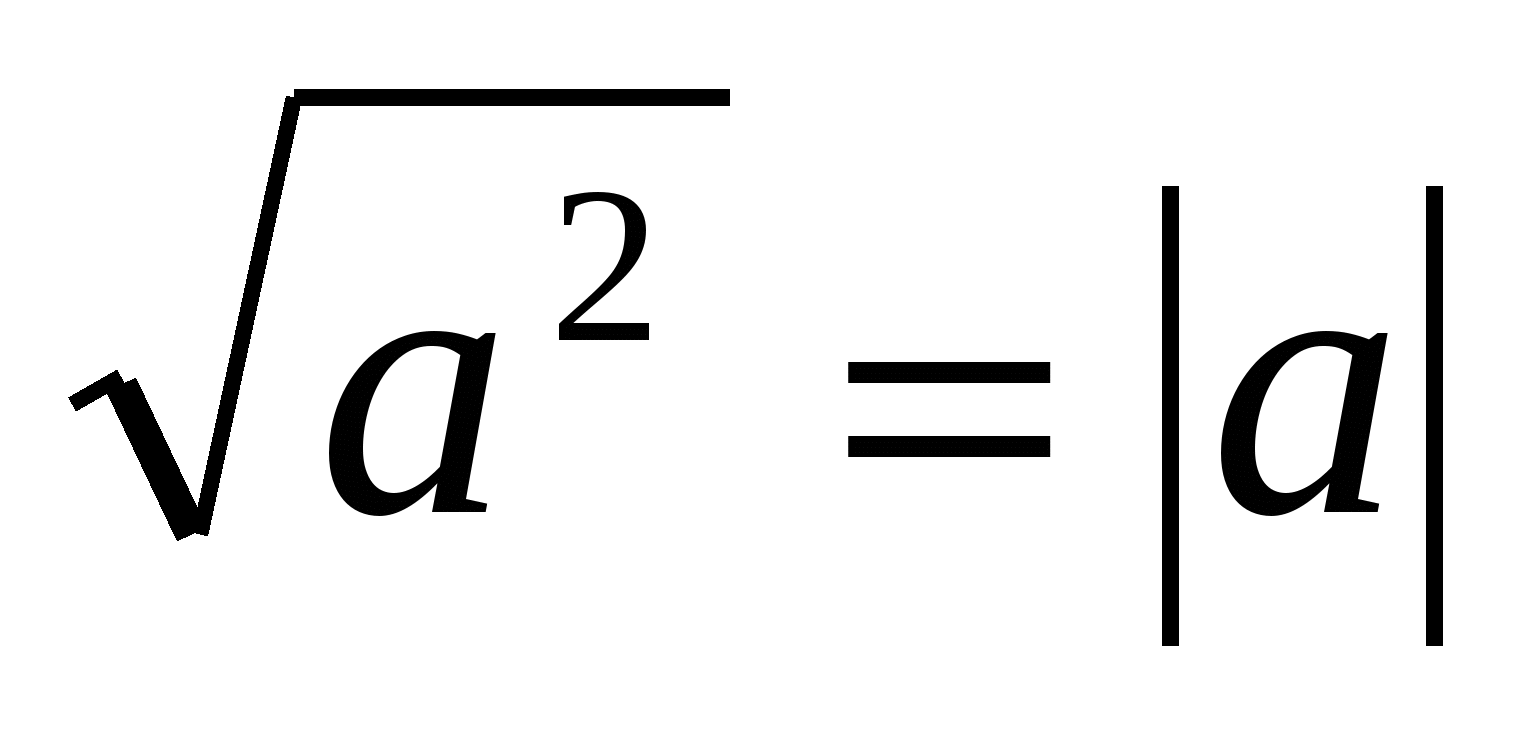
, since it is used not only for solving equations and inequalities, but also for transforming many expressions with radicals. The rest of the solution methods that we have considered, of course, are of great interest in terms of expanding the mathematical horizons and general mathematical development. Therefore, we plan to use them to prepare for the state final attestation in the form of the Unified State Examination and preparation for studying at a higher educational institution.

Bibliography.

"Great Mathematical Encyclopedia" for schoolchildren and students;

Mathematics. USE - 2011, 2012. Standard examination options. / Edited by A.L. Semenova, I.V. Yashchenko.

M.Ya. Vygodsky. Handbook of Elementary Mathematics

"The newest student reference book";

Encyclopedia “I know the world. Mathematics";

;